- About MAA
- Membership
- MAA Publications
- Periodicals
- Blogs
- MAA Book Series
- MAA Press (an imprint of the AMS)
- MAA Notes
- MAA Reviews
- Mathematical Communication
- Information for Libraries
- Author Resources
- Advertise with MAA
- Meetings
- Competitions
- Programs
- Communities
- MAA Sections
- SIGMAA
- MAA Connect
- Students
- MAA Awards
- Awards Booklets
- Writing Awards
- Teaching Awards
- Service Awards
- Research Awards
- Lecture Awards
- Putnam Competition Individual and Team Winners
- D. E. Shaw Group AMC 8 Awards & Certificates
- Maryam Mirzakhani AMC 10 A Awards & Certificates
- Two Sigma AMC 10 B Awards & Certificates
- Jane Street AMC 12 A Awards & Certificates
- Akamai AMC 12 B Awards & Certificates
- High School Teachers
- News
You are here
Moses ibn Tibbon’s Hebrew Translation of al-Hassar's Kitab al Bayan - Denomination
Al-Hassar’s Kitāb al-Bayān is a didactic text; it addresses the student directly and is written in a clear and user-friendly style. The worked examples all assume the student is working with a dust board. The methods of calculation require moving numbers around and rubbing out some as the work proceeds, a mode for which the dust board was well suited in the same way as are a black or white board, chalk or marker, and eraser (see Note 1). For these methods, finding the result was what mattered and not the intermediary steps. However, some algorithms suitable for paper and pen or pencil calculations appear in the appendices of both the Christ Church and Vatican manuscripts and therefore are possibly from as early as the 14th century. These algorithms are described in the last webpage of this article.
In his Introduction, al-Hassar states that he has arranged the work in two parts: the first on integers and the second on fractions. For our descriptive purposes, however, we will divide the text into three parts: Part One on integers; Part Two on fractions; Part Three on computations (see Note 2). By reason of its superior physical condition, the Christ Church manuscript will be our primary Hebrew source.
In Part One, integers and the operations associated with them are examined under ten chapter headings (see Note 3):
- Numeration: On number scales (levels) and number names
- Notation: The Gobar digits and their use in a positional decimal notation
- Addition
- Subtraction
- Multiplication
- Denomination
- Division
- Halving
- Doubling
- Extraction of Roots (see Note 4).
The chapter on Denomination is subtitled “Division of a Small Number by a Larger One,” and it is here that the horizontal bar notation first appears in the context of the naming and symbolic representation of the ratio of a smaller number to a larger one using Gobar numerals (integers). The chapter on Multiplication is followed by that on Denomination and not, as might have been expected, by that on Division, because the new notation would be required in the latter.
The chapter on Denomination opens with a review of the various classes of integers – prime or composite, odd or even – and of their divisibility and remainder rules. Division is treated as the repeated subtraction (השלכה) of the divisor (divider) from the dividend. Following this preamble, which al-Hassar states is an essential prerequisite to the subject at hand, the formal classical definition of the ratio of a smaller number to a larger one is presented:
The ratio of a smaller number to a larger one is the number of parts, one or more, that the former is of the latter.
This definition echoes that of Euclid: “Each number is either a part of a larger number [a unit fraction] or parts [a proper fraction] of it” (Elements VII.4). Al-Hassar obtains from it the following names (designations):
[Thus]…the ratio of one to three is called “a third” [one part of three] … that of one to four, “a fourth” [one part of four]; that of two to four, “two fourths" [two parts of four] … that of six to eight, “six eighths” [six parts of eight] … that of nine to ten, “nine tenths” [nine parts of ten].
Thus far, it is all quite straightforward and familiar. But al-Hassar now presents a different method for naming the ratios in those instances where the larger number can be factorized.
When it is said to you, “Name one part of fifteen.” Now you have already learned that fifteen is a composite number that arises from the multiplication of three by five; it follows, therefore, that three is one fifth of fifteen and five is one third of fifteen; thus, since one is a third of three, one part of fifteen [a fifteenth] is “one third of a fifth” \(\left[{\frac{1}{3}} \cdot{\frac{1}{5}}={\frac{1}{15}}\right]\).
Based on this example, al-Hassar proposes that seven parts of fifteen [seven fifteenths], might be symbolically represented by integers as follows:
Write the factors \(3\) and \(5\) in a line, and put the \(7\) over the \(3\):
\[\genfrac{}{}{0pt}{}{7\quad \phantom{x}}{3\quad 5}\]
Now find a multiple of \(3\) which, when deducted from \(7,\) leaves a remainder less than \(3;\) the only possibility here is multiplier \(2\) and remainder \(1\) \([7-(2\times 3) = 1].\)
Delete the seven and replace it by the remainder, \(1;\) put the multiplier, \(2,\) over the \(5\):
\[\genfrac{}{}{0pt}{}{1\quad {2}}{3\quad 5}\]
Now draw a line between the two rows of numbers: \[\frac{1\quad 2}{3\quad 5}\]
Al-Hassar reads this symbolic representation, from right to left, as “two fifths and a third of a fifth” (see Note 5):
\[\frac{1\quad 2}{3\quad 5}\implies\frac{2}{5}+\frac{1}{3\times 5}=\frac{7}{15}\]
He derives in a similar way a symbolic representation for eleven fifteenths: \[\frac{2\quad 3}{3\quad 5}\] He reads this notation, from right to left, as “three fifths and two thirds of a fifth”: \[\frac{2\quad 3}{3\quad 5}\implies\frac{3}{5}+\frac{2}{3\times 5}=\frac{11}{15}\] As we will see, whereas multi-digit integers are read from left to right in the direction of decreasing powers of ten, the symbolic representation of fractions is written and read from right to left.
In modern terminology, what al-Hassar contrived was a composite fraction notation in which a sequence of numerators and denominators are aligned, one above the other, with a horizontal line between them. Reading from right to left, each of the successive terms above the line is the numerator of the fraction whose denominator is the product of all the terms below and to the right of it. In the simplest case – two integers, \(a\) and \(b,\) above the line and two, \(c\) and \(d,\) below – this gives:
\[\frac{b\quad a}{d\quad c}\implies \frac{a}{c}+\frac{b}{dc}.\]
Employing this notation, a fifteenth is denoted in the Christ Church Library manuscript by
 |
\[\frac{1\quad\phantom{0}}{3\quad 5}\implies \frac{0}{5}+\frac{1}{3\times 5}=\frac{1}{15},\] |
seven fifteenths by
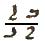 |
\[\frac{1\quad 2}{3\quad 5}\implies \frac{2}{5}+\frac{1}{3\times 5}=\frac{7}{15},\] |
and eleven fifteenths by
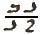 |
\[\frac{2\quad 3}{3\quad 5}\implies \frac{3}{5}+\frac{2}{3\times 5}=\frac{11}{15}.\] |
The copyist of the Christ Church manuscript chose to place the symbolic representations in the margins outside the running text, whereas they are embedded within the text in the Vatican manuscript (see Figure 8, below).

Figure 8. Folio 5r of the Christ Church manuscript. Reading from top to bottom, the three entries in the margin between the columns are:
\[\frac{1\quad 2}{3\quad 5}\implies \frac{7}{15},\quad\quad \frac{2\quad 3}{3\quad 5}\implies \frac{11}{15},\]
and
\[\frac{1\quad 0\quad 0}{2\quad 6\quad 8}\implies \frac{0}{8}+\frac{0}{6\times 8}+\frac{1}{2\times 6\times 8}=\frac{1}{96}\]
As shown by this last example, the notation can be expanded to more terms via formulas of the form \[\frac{c\quad b\quad a}{f\quad e\quad d}\implies\frac{a}{d}+\frac{b}{ed}+\frac{c}{fed}\] and so on. (Image used by permission of Christ Church College Library)
Al-Hassar's novel composite fraction notation was later taken up by Fibonacci (c. 1175-1250) in Chapter 5 of his Liber Abaci (1202). This notation also appears in a work by the Maghreb mathematician ibn al Yasamin (d. 1204), Talqih al-afkar bi rushum huruf al-ghubar, or Fertilization of Thoughts with the Help of Dust Letters (Lamrabet). However, it would take another three centuries for fraction notation to evolve into the simpler notation now commonly used for all vulgar (common) fractions.
Note 1. According to A. S. Saidan, “Hindu arithmetic entered Islam with the dust board (takht) as an intrinsic tool of it, writing and rubbing out being made by the fingers or with a stylus” (p. 351). There is no clear evidence, however, for use of the dust board in India.
Note 2. In his translation of the Gotha manuscript, Suter (1901) divides the treatise into seven Chapters (Kapiteln): our Parts 1 and 2 correspond to his Chapters 1 and 2; our Part 3 encompasses his Chapters 3 to 7. The Gotha manuscript does not have the appendices found in the two Hebrew manuscripts.
Note 3. Folios 1r to 7r in the Christ Church manuscript and folios 1r to 9v, 17r to 17v and 10r to 14r (in that order), in the Vatican manuscript
Note 4. This chapter only deals with the roots of “square” numbers, e.g. 625 (sq. rt. = 25) and 583696 (sq. rt. = 764). Extracting the square roots of integers and fractions in general is the final topic in al-Hassar’s treatise; it appears in folios 30r to 31v in the Christ Church codex, folios 69v to 73r in the Vatican manuscript, and pages 37 to 39 (Siebentes Kapitel) in Suter’s translation of the Gotha manuscript.
Note 5. Henceforth, the symbol \(\implies\) will indicate a replacement of al-Hassar's representation of arithmetic operations by modern notation.
Jeremy I. Pfeffer (Hebrew University of Jerusalem), "Moses ibn Tibbon’s Hebrew Translation of al-Hassar's Kitab al Bayan - Denomination," Convergence (May 2017)




