- About MAA
- Membership
- MAA Publications
- Periodicals
- Blogs
- MAA Book Series
- MAA Press (an imprint of the AMS)
- MAA Notes
- MAA Reviews
- Mathematical Communication
- Information for Libraries
- Author Resources
- Advertise with MAA
- Meetings
- Competitions
- Programs
- Communities
- MAA Sections
- SIGMAA
- MAA Connect
- Students
- MAA Awards
- Awards Booklets
- Writing Awards
- Teaching Awards
- Service Awards
- Research Awards
- Lecture Awards
- Putnam Competition Individual and Team Winners
- D. E. Shaw Group AMC 8 Awards & Certificates
- Maryam Mirzakhani AMC 10 A Awards & Certificates
- Two Sigma AMC 10 B Awards & Certificates
- Jane Street AMC 12 A Awards & Certificates
- Akamai AMC 12 B Awards & Certificates
- High School Teachers
- News
You are here
Kepler: The Volume of a Wine Barrel - Links and References
Links
 |
Kepler in MacTutor History of Mathematics Archive A biography of Johannes Kepler (1571-1630) |
 |
Original Latin version of Kepler's Nova stereometria (Posner Memorial Collection, Carnegie Mellon University Libraries) Kepler's German version of his Nova stereometria (two pages, Loci: Convergence Mathematical Treasures) |
 |
Kepler in MatematicasVisuales - The Area of a Circle Kepler's intuitive infinitesimal approach to determine the area of a circle |
 |
Kepler's intuitive infinitesimal approach to determine the volume of a sphere |
 |
Two solids of revolution defined by Kepler |
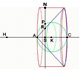 |
Archimedes' Method for Computing Areas and Volumes Article by Gabriela Sanchis in Loci: Convergence explaining Archimedes' Method based on the Law of the Lever |
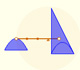 |
Archimedes in MatematicasVisuales - Area of a Parabolic Segment Archimedes used infinitesimal methods to discover areas and volumes 1800 years before Kepler and Cavalieri. |
 |
The Sagacity of Circles: A History of the Isoperimetric Problem Article by Jennifer Wiegert in Loci: Convergence summarizing the history of the problem of finding the region of greatest area bounded by a given perimeter
|
 |
Thinking Outside the Box - or Maybe Just About the Box Article by David Meel and Thomas Hern in Loci presenting a box optimization problem more realistic and hence more complex than the standard one. |
References
Margaret E. Baron, The Origins of the Infinitesimal Calculus, Dover Publications, New York, 1987; originally published in 1969 by Pergamon Press, Oxford, England.
Carl B. Boyer, The History of the Calculus and its Conceptual Development, Dover Publications, New York, 1959, reprint of 1949 edition of Boyer's The Concepts of the Calculus: A Critical and Historical Discussion of the Derivative and the Integral, Hafner Publishing, originally published in 1939.
Roberto Cardil, Kepler: The Best Proportions for a Wine Barrel (interactive applet), MatematicasVisuales, posted 2009-2010.
Roberto Cardil, Kepler: The Volume of a Wine Barrel (interactive applet), MatematicasVisuales, posted 8 January 2010.
Roberto Cardil, "Kepler: The Volume of a Wine Barrel" (copy of this article with four interactive applets), MatematicasVisuales, posted 2011
Roberto Cardil, MatematicasVisuales: www.matematicasvisuales.com
C.H. Edwards, The Historical Development of the Calculus, Springer-Verlag, New York, 1979.
Kepler, Johannes, Nova stereometria doliorum vinariorum, Linz, 1615, held in the Posner Memorial Collection of the Carnegie Mellon University Libraries, Pittsburgh, Pennsylvania, call number 520 K38PN, available in electronic format: http://posner.library.cmu.edu/Posner/books/book.cgi?call=520_K38PN
Felix Klein, Elementary Mathematics from an Advanced Standpoint - Arithmetic - Algebra - Analysis (especially pp. 207-210), Dover Publications, New York, 2004; originally published in 1908 in Leipzig.
Dirk J. Struik (ed.), A Source Book in Mathematics, 1200-1800 (see pp. 192-197: "Kepler. Integration Methods"), Harvard University Press, Cambridge, MA, 1969.
Otto Toeplitz, The Calculus: A Genetic Approach (especially pp. 80-83), University Of Chicago Press, 1963.
Roberto Cardil (MatematicasVisuales), "Kepler: The Volume of a Wine Barrel - Links and References," Convergence (January 2012), DOI:10.4169/loci003499




