- About MAA
- Membership
- MAA Publications
- Periodicals
- Blogs
- MAA Book Series
- MAA Press (an imprint of the AMS)
- MAA Notes
- MAA Reviews
- Mathematical Communication
- Information for Libraries
- Author Resources
- Advertise with MAA
- Meetings
- Competitions
- Programs
- Communities
- MAA Sections
- SIGMAA
- MAA Connect
- Students
- MAA Awards
- Awards Booklets
- Writing Awards
- Teaching Awards
- Service Awards
- Research Awards
- Lecture Awards
- Putnam Competition Individual and Team Winners
- D. E. Shaw Group AMC 8 Awards & Certificates
- Maryam Mirzakhani AMC 10 A Awards & Certificates
- Two Sigma AMC 10 B Awards & Certificates
- Jane Street AMC 12 A Awards & Certificates
- Akamai AMC 12 B Awards & Certificates
- High School Teachers
- News
You are here
Helping Ada Lovelace with her Homework: Classroom Exercises from a Victorian Calculus Course – Lovelace’s Early Mathematical Education
Ada’s earliest introduction to mathematics instruction appears to have occurred around the age of five. A notebook kept by her governess, Miss Lamont, records that she ‘Commenced giving instructions to Miss Byron’ in May 1821. As Miss Lamont reported to Ada’s mother Lady Byron: ‘The first trial was in arithmetic. She adds up sums of five or six rows of figures, with accuracy; she is deliberate and correct in the process, and takes an interest in the performance’ [LB 118, item 5, 14 May 1821, f. 2r]. By the age of ten, Ada had been introduced to more advanced arithmetical techniques, such as the so-called Rule of Three, as illustrated in a letter to her mother, in which she wrote:
I have been puzzling hard at a sum in the rule of three which I could not do, the question is “If 750 men are allowed 22500 rations of bread per month, how many rations will a garrison of 1200 men require"?’ [LB 41, 1 June 1826, ff. 27r-27v].

Figure 3. Page from John Bonnycastle’s An Introduction to Arithmetic (1810) featuring Ada’s Rule of Three problem.
This type of problem, while relatively trivial today, was considered quite challenging at the time and was very common in 18th- and 19th-century mathematical textbooks. It was also considered to be a branch of arithmetic, whereas today it would be dealt with purely algebraically. Given three known numbers, \(a\), \(b\), and \(c\), the Rule of Three says that, if \[\frac{a}{b}=\frac{c}{x},\] then \[x=\frac{bc}{a} .\]
As can be seen from the extract from a contemporary 19th-century textbook above, the answer to Ada’s problem is 36,000 rations of bread. You will find a fuller explanation in the Solutions section of this article.
From this and other letters between mother and daughter, it would appear that Lady Byron was partly responsible for some of Ada’s initial education in mathematics, as she had herself received tuition in mathematics and science in her childhood, which was unusual for young ladies at the time. In particular, we see that she corrected her 13-year-old daughter’s misunderstandings concerning order of operations, from a passage in which Ada notes that (5×2)+(3×4)=22, and not 52 as she had previously believed:
I had not till Mama showed me, understood the sums where both multiplication and addition signs were used, in consequence of which the former examples must have been wrong, as I thought they were as follows: 5×2=10 to which add 3 = 13 which multiplied by 4 gives 52, instead of 5×2=10 to which add 3×4=12 [LB 175, 10 Feb. 1829, f. 176r].
By the age of 18, Ada was receiving informal guidance on her mathematical studies from an old family friend, Dr. William King, who had graduated from Cambridge a quarter of a century before. At his suggestion, she began working through Euclid’s Elements, writing to him that she was covering about ‘four new propositions a day, and go[ing] over some of the old ones. I expect now to finish the 1st book in less than a week’ [LB 172, 24 March 1834, f. 131r]. Before long, she was conjecturing her own variation of the Pythagorean theorem:
Can it be proved . . . that equilateral triangles being constructed on the sides of a right angled triangle, and also one on the hypotenuse, the sum of the triangles on the sides is equal to the triangle on the hypotenuse? . . . It strikes me that it ought to be as demonstrable as when the figures are four-sided & equilateral. [LB 172, Ada Byron to Dr King, 13 April 1834, f. 132r]
(You can see a sketch of roughly what she had in mind here.) But Dr. King was long out of practice with this sort of mathematics and unable to help her: ‘You will soon puzzle me in your studies,’ he replied [LB 172, 24 April 1834, f. 133r]. In just a few weeks, Ada had reached the bounds of her tutor’s expertise.
Around the time of her marriage to William, the 8th Lord King (who became the Earl of Lovelace in 1838), Ada turned for mathematical assistance to a new friend, Mary Somerville (1780–1872). In addition to being a respected and well-known scientific author, Somerville was acquainted with more up-to-date analytical methods, then comparatively new to British mathematics. By 1835, shortly after her marriage, Ada was reporting to Somerville that her mathematical reading had progressed to trigonometry and algebra: ‘I now read Mathematics every day & am occupied in Trigonometry & in preliminaries to Cubic & Biquadratic Equations. So you see that matrimony has by no means lessened my taste for those pursuits, nor my determination to carry them on...’ [Somerville Papers, Dep. c.367, Folder MSBY-3, 1 Nov. 1835, f. 55v].

Figure 4. Mary Somerville (1780–1872) in 1834, by Thomas Phillips. National Galleries Scotland.
But despite this apparent progress, her correspondence with Mary Somerville reveals significant gaps in the mathematical knowledge and abilities of the twenty-year-old Ada. In the same letter of 1835, she recounted a difficulty she was experiencing in trigonometry, namely to use the addition formulae
\[\sin(a+b)=\frac{\sin a \cos b+\sin b\cos a}{R}\]
\[\cos(a+b)=\frac{\cos a \cos b - \sin a\sin b}{R}\]
to derive expressions for \(\sin (a-b)\) and \(\cos (a-b)\). Of course, as Somerville was able to tell her, since \(\sin(-b) = - \sin \)b and \(\cos(-b)=\cos b\), the solution was simply a matter of substituting \(-b\) for \(b\) in the above formulae [LB 174, 8 Nov. 1835, ff. 29r-29v]. (Note that the use of a general radius of length R not necessarily equal to 1, such that \(sin^2 x+cos^2 x=R^2\), was not uncommon in trigonometry textbooks at this time.)
But the matter did not end there. Two weeks later, Ada was asking a similar, and only slightly harder Trigonometry Problem, namely, how to use
Once again, Somerville was able to resolve the difficulty in a few lines. If you wish to see how she solved the problem, click here.
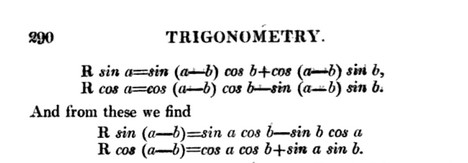
Figure 5. Extract from the translation of Legendre’s Elements of Geometry and Trigonometry
published by David Brewster (1822), with which Ada Lovelace had difficulty.
Between 1836 and 1839, Ada gave birth to three children, which interrupted her mathematical studies somewhat, but in late 1839, after the birth of her third child, she began actively looking for a new tutor. By the summer of 1840, she could report to her mother that a suitable tutor had been found and her mathematical studies were once more underway. That tutor was Augustus De Morgan.
Adrian Rice (Randolph-Macon College), "Helping Ada Lovelace with her Homework: Classroom Exercises from a Victorian Calculus Course – Lovelace’s Early Mathematical Education," Convergence (September 2021)




