- About MAA
- Membership
- MAA Publications
- Periodicals
- Blogs
- MAA Book Series
- MAA Press (an imprint of the AMS)
- MAA Notes
- MAA Reviews
- Mathematical Communication
- Information for Libraries
- Author Resources
- Advertise with MAA
- Meetings
- Competitions
- Programs
- Communities
- MAA Sections
- SIGMAA
- MAA Connect
- Students
- MAA Awards
- Awards Booklets
- Writing Awards
- Teaching Awards
- Service Awards
- Research Awards
- Lecture Awards
- Putnam Competition Individual and Team Winners
- D. E. Shaw Group AMC 8 Awards & Certificates
- Maryam Mirzakhani AMC 10 A Awards & Certificates
- Two Sigma AMC 10 B Awards & Certificates
- Jane Street AMC 12 A Awards & Certificates
- Akamai AMC 12 B Awards & Certificates
- High School Teachers
- News
You are here
Mirror Image Symmetry from Different Viewpoints
Insights and techniques from mathematics can do much to illuminate the workings of the physical world; they can play key roles in the understanding of molecular structures crucial to life.
Speaking at the MAA Carriage House on September 22, Erica Flapan (Pomona College) sandwiched chemistry between the mathematical fields of geometry and topology. In “Mirror Image Symmetry from Different Viewpoints,” Flapan underscored the importance of precise definitions, found humor in the pharmaceutical industry, and showed that even seemingly purest-of-pure mathematics may prove applicable to real-world problems.
Flapan established early that mirror image symmetry matters for reasons beyond the aesthetic. Even as we yearn for that perfectly balanced face we’re told will boost our attractiveness, we are, at a microscopic level, fundamentally asymmetric.
“All biological things—like us—are made up of oriented molecules,” Flapan said. Our amino acids all spiral in one direction, she explained, and are different from their mirror images. Just as a lefty reacts differently to a right-handed desk than a left-handed one, so an asymmetric amino acid reacts differently to two molecules that are non-identical mirror images of each other.
Take the painkiller Darvon. Its mirror image acts as a cough suppressant, which drugmaker Eli Lilly marketed as Novrad. Notice anything about the names of the two medications?
“Even a pharmaceutical company can have a sense of humor when it comes to mirror image symmetry,” Flapan quipped.
Asymmetric molecules don’t always bless Big Pharma with lucrative two-for-ones, though. The mirror reflection of the anti-inflammatory Naproxen is toxic. Synthesis typically results in a 50-50 mix of the two varieties, and separating the helpful molecules from the harmful ones costs drugmakers time and money.
So with life, death, and mega-millions riding on it, what exactly is mirror image symmetry? Chemists use the word chiral to talk about the symmetry of molecules. “Chiral” comes from the Greek for “hand,” so a molecule that is chiral is, like a hand, different from its mirror image. An achiral molecule is not like a hand; it is the same as its mirror image.
Chemistry is slippery business, but geometry—the study of rigid objects—less so. Flapan defined a molecule as geometrically chiral if it cannot be rigidly superimposed on its mirror image. Easy enough to show.
But chemists care more about behavior in solution than freeze-frame looks, and many molecules are not the rigid objects of geometry. They have moveable parts or become increasingly flexible when heated. A molecule is chemically chiral, then, if it cannot transform into its mirror image.
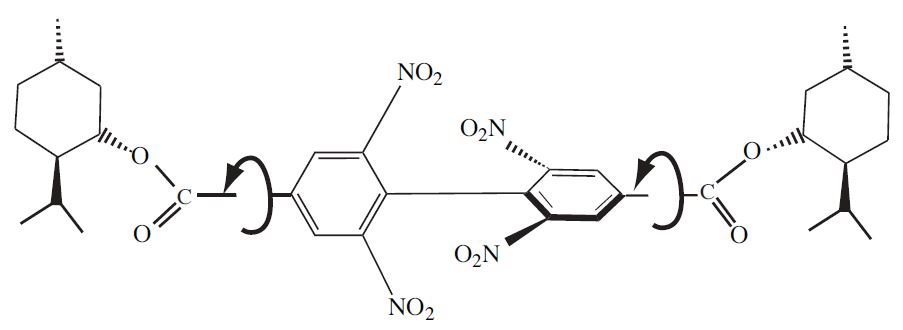
Kurt Mislow's molecule is geometrically chiral but chemically achiral.
Chemists often use plain “chiral” for both geometrically chiral and chemically chiral, but Flapan emphasized the need for the distinction. She walked her Carriage House audience through the (chemically possible) transformation of a geometrically chiral molecule into its mirror image. The molecule, synthesized in the 1950s by Kurt Mislow expressly to establish the difference between geometric and chemical chirality, has two simultaneously rotating propeller-like appendages.
Jumping to the extreme opposite of geometry’s rigidity, Flapan defined a molecule as topologically chiral if it cannot be deformed to its mirror image assuming complete flexibility. As an example of a molecule that is topologically and chemically achiral, but geometrically chiral, she offered a molecule modeled by two interlocking individually asymmetric rings.
If a molecule cannot be deformed topologically into its mirror image, Flapan noted, it will not be able to chemically transform into its mirror image either. Heat a geometrically chiral molecule enough, Flapan said, and you might be able to force it into its mirror image form. Not so with a topologically chiral molecule, though. The modifications prohibited in topology—an object passing through itself, for instance—also don’t fly in chemistry.
“The concept of topological chirality not only is useful in proving certain molecules are chiral,” said Flapan, “but it’s useful in telling us they’ll remain chiral [even when heated]. So it has a further importance in chemistry.”
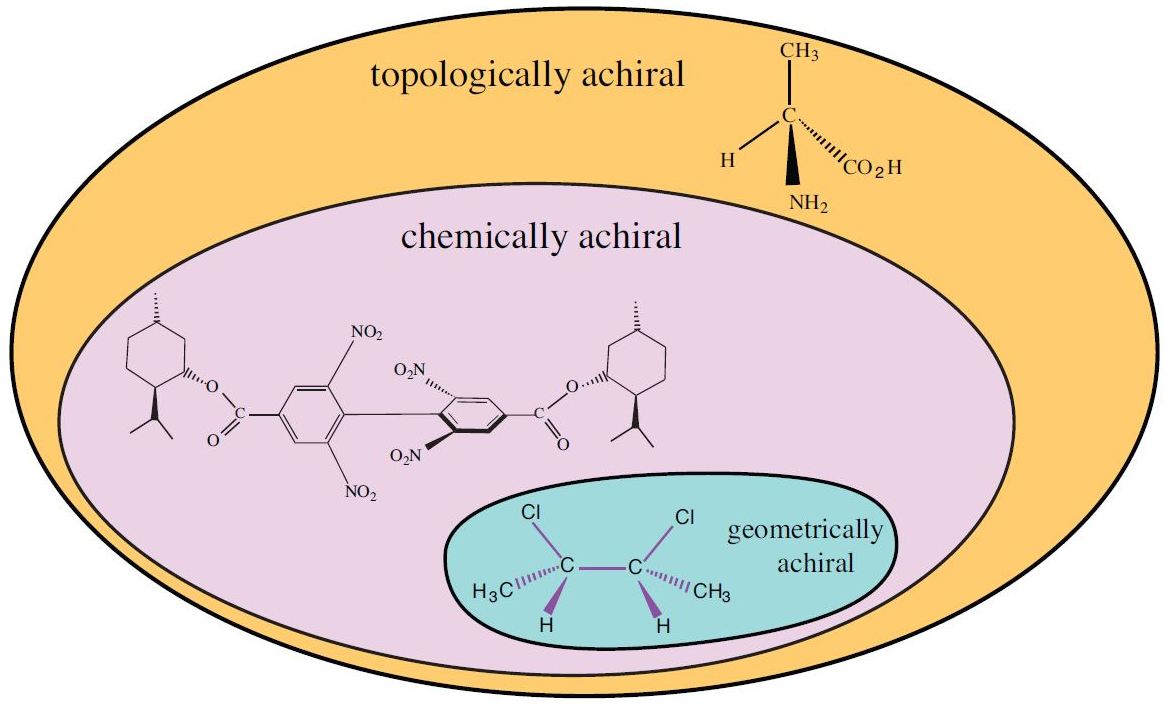
A set diagram showing the relationship between the different sorts of chirality
Flapan captured the relationship between the different sorts of chirality with a set diagram comprised of three nested ovals. The set of geometrically achiral (achiral=symmetric, remember) molecules is the smallest, but all geometrically achiral molecules are chemically achiral and all chemically achiral molecules are topologically achiral.
The characteristic we care about, then—chemical chirality—is wedged between the two mathematical conceptions of chirality, not precisely characterized by either.
Nonetheless, topological chirality is a useful concept for chemists. Since the publication of her book When Topology Meets Chemistry: A Topological Look at Molecular Chirality, Flapan has been contacted by chemists inquiring about the topological chirality of molecules they’re studying. Given the demand for such information, topologists like Flapan adapt old tools—and develop new ones—to address these questions of molecular symmetry.
“So a lot of topology, which was originally thought of as being very pure, turned out to be useful,” Flapan said. —Katharine Merow
Watch a short version of the lecture on YouTube.
 Listen to audio of Erica Flapan's lecture (mp3)
Listen to audio of Erica Flapan's lecture (mp3)
 Listen to an interview with Erica Flapan and MAA Director of Publications Ivars Peterson (mp3)
Listen to an interview with Erica Flapan and MAA Director of Publications Ivars Peterson (mp3)

This MAA Distinguished Lecture was funded by the National Security Agency.




