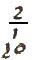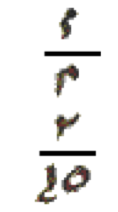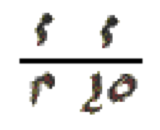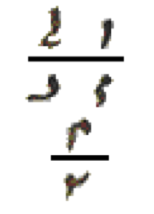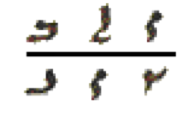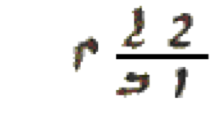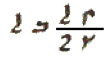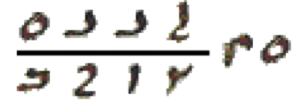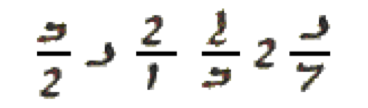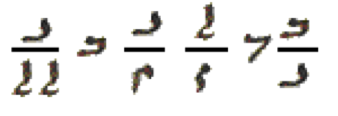The earliest known external reference to a mathematical opus by Abu Bakr Muhammad ibn Abdallah ibn Ayyash al-Hassar appears in ibn Khaldun’s 14th century work Muqaddimah (see Note 1), where he refers to it as “the little al-Hassar.” Five centuries were to pass, however, before an actual copy of al-Hassar’s Kitāb al-Bayān wa-l-tadhkār was discovered and then only in the guise of a Hebrew translation. It was Moritz Steinschneider who, in 1874, first made the connection between “the little al-Hassar” cited by ibn Khaldun and a Hebrew manuscript of a mathematical treatise by Abu Bakr al-Hassar in the Vatican Library (Steinschneider, pp. 557-558). Six years later, Adolf Neubauer, a librarian at the Bodleian Library, Oxford, identified a second copy of the same Hebrew translation at the Christ Church Library, Oxford. A third Hebrew version of al-Hassar’s treatise has surfaced more recently as one of the works in a composite codex at the Russian State Library, Moscow.

Only in 1893 was an Arabic manuscript of al-Hassar’s Kitāb al-Bayān first found and identified by W. Pertsch among the Arabic manuscripts in the Gotha Library, University of Erfuth (Ms. 1489, noted in Pertsch, p. 114). In 1901, the mathematician Heinrich Suter translated extracts from this manuscript into German in the course of his extensive study of medieval Arabic mathematical texts (Suter 1901, pp. 12-40). There is no copyist’s colophon as such but the last line in the manuscript reads [in translation]:

The blessed book is finished, praise be to Allah, for his help and favour … on Tuesday, 13^th Muḥarram 836 [September 9, 1432].

The existence of a further six Arabic manuscripts containing all or parts of the Kitāb al-Bayān has since been reported, making a total of seven copies in all. The oldest of these is in the Lawrence J. Schoenberg Collection at the University of Pennsylvania. Written in Baghdad and dated Safar 590 [January/February 1194], it lacks the last quarter of the text of the Gotha manuscript (see Note 2). The most recently uncovered copy is a 16th century manuscript sold at auction in London in 2016. The remaining five Arabic manuscripts are cited in two articles by Paul Kunitzsch (2002-03 and undated).

The Vatican Library, Rome, manuscript

The colophon in the Vatican manuscript (folio 76v) gives the name of the copyist as Baruch b. Solomon b. Joab, or, in Hebrew, ברוך הבי"א [הצעיר בבית אבי] ב"ר שלמה אבי ישר"ו ב"ר יואב ישר"ו, and the date and place of its completion as Thursday, 25th Shevat 5211 [February 6, 1451] and Montalcino, Italy; it does not, however, name the translator from the original Arabic. The best match to the partial watermarks clearly visible in the paper is Briquet 6645, which, if correct, would indicate that the paper was also used for documents created or found in Lucca, Italy, in 1445, according to Charles Briquet’s Les Filigranes. The manuscript is bound in a codex measuring 21 x 15 cm. which has the insignias of Pope Urbanus VIII (who held the office of Pope from 1623 to 1644) and of his nephew and librarian Francisco Barberini (1626-1633) embossed in gold onto its green front and back covers, respectively. Thus, the binding evidently dates from some time around 1630. There are, however, clear indications that the folios were severely cropped, both top and bottom, at the time. The original length of the pages, at least 22 cm., can be gauged from fol. 16v which has a long marginal note starting down the right hand side and continuing across the page below the main text. Cropping the bottom of the folio to the same length as the other folios would have meant losing all or part of the last three lines of the marginal note. To avoid this, the conscientious binders folded over the bottom centimetre of the folio instead of cutting it off! The outer spine of the codex was replaced in the 19th century and bears the insignias of Pope Pius IX (1846-1878) and his librarian Angelus Mai (1853-1854).

Notwithstanding, the codex is unfortunately not in the best of condition. The binding has broken open in a number of places and folios have come loose. Furthermore, in two instances the foliation does not correspond to the flow of the text: the folios numbered 17 and 19 should actually follow folio 9 leaving just folio 18 between folios 16 and 20 (see Note 3). It would appear that the foliation predates the binding in 1630, for in numerous instances the cropping cuts straight through the folio numbers in the top left-hand corner of the recto pages. The said folios were apparently already out of place even before the manuscript was bound, or more likely rebound, into the present codex around 1630.

The Christ Church Library, Oxford, manuscript

By contrast, the Christ Church manuscript (to which we will often refer as codex 189) is in almost mint condition. Measuring 30 x 23 cm., it comprises a total of 77 folios: 43 blank sheets of coarse paper (no watermark) – six at the front and 37 at the back – and 34 folios of watermarked paper sandwiched between them on which the Hebrew text is written (see Figure 1). The script is Provencal/Sefardic cursive and the text is configured in a single column on fols. 1r to 4v and double columns from fol. 5r to fol. 33r. The purpose of the 43 blank sheets is unclear.

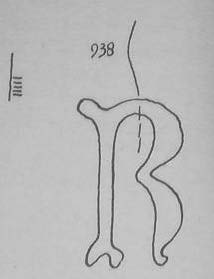
The watermark in the Christ Church codex 189	Briquet 8941	Zonghi 938 (Italy 1456)

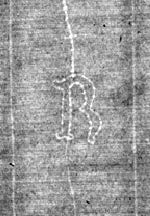
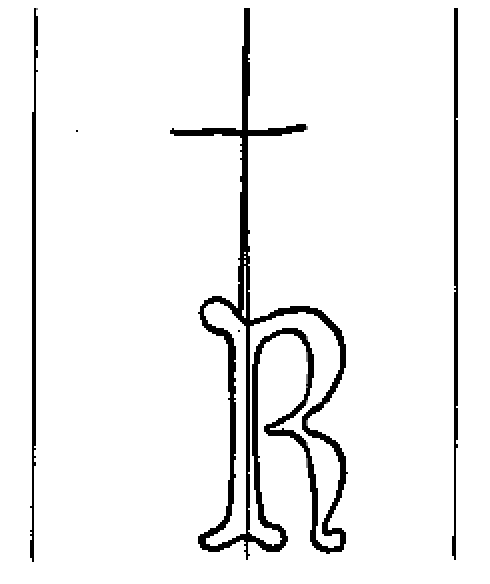
Figure 1. The watermark in the 34 folios in the Christ Church codex on which Moses ibn Tibbon’s Hebrew translation is written (left) is compared with two possible watermarks of the era. Paper with the watermark labeled "Briquet 8941" (middle) was used for documents created or found in Palermo in 1467, Bavière 1470, Naples 1470, Amalfi 1471, and Catania 1472, according to Charles Briquet’s Les Filigranes (p. 478). Alternatively, documents on paper with the watermark "Zonghi 938" (right) were created or found in Italy in 1456, according to Zonghi’s Watermarks. All of these dates are consistent with those in the copyist’s colophon.

 

Figure 2. Fol. 31v of codex 189, Christ Church College Library. Note the two colophons at the foot of the right-hand column, that of the translator, Moses bar Shmuel Tibbon, and, below it, that of the unnamed copyist. Hebrew is read right to left, top to bottom. The lefthand column contains the start of an appendix to al-Hassar's Kitāb al-Bayān consisting of nine additional worked examples that do not appear in any of the extant Arabic manuscripts. The Hebrew translation was evidently quite widely dispersed amongst the Jews of Provence and Italy and these additional examples, which will be discussed in the final webpage of this article, are indicative of the move from the sandboard to paper-and-ink as the knowledge of arithmetic spread into Europe, a transition without which mathematics as a whole could not have developed as it did. (Image used by permission of Christ Church College Library)

As shown in Figure 2, above, there are two colophons in the righthand column of fol. 31v. The first is that of Moses ibn Tibbon, the translator into Hebrew of the Arabic original:

The work is done; and it is the Book of Arithmetic by Abu Bakr Mohammad, son of Abdullah, son of Abbas al-Hassar; and R. Moses ben R. Shmuel [ibn Tibbon], ben R. Yehudah, ben R. Shaul of blessed memory from Ramon Sefarad (Spain), translated it; and its translation was completed on the 18th day of the month of Iyar in the year 31 [May 12, 1271] in the city of Montpelier.

The second colophon is that of the manuscript’s copyist:

And here Bari[?] (והנה בארי), its transcription was completed on the 12^th day of the month of Adar Bet in the year 236 of the sixth millennium [March 17, 1476], one thousand four hundred and eight years since the destruction of the Temple, may it be speedily rebuilt, Amen Selah.

The copyist is not named. In order to discuss possible alternatives for "Bari," we magnify the copyist's colophon (see Figure 3).

Figure 3. The copyist’s colophon in the Christ Church College manuscript reads: “… its transcription was completed ... one thousand four hundred and eight years after the destruction of the Temple.” A note below in a darker ink reads: “The Temple was destroyed 68 years after the incarnation (נחרב הבית ס"ח שנים אחר ההגשמה).” Adding these 68 years from the birth of Jesus also gives 1476 AD for the year the manuscript was written. (Image used by permission of Christ Church College Library)

There is a copyist’s mark, ד', above the word בארי in the top line of the colophon (see Figure 3). Its purpose appears to be to indicate that the word is unusual or exceptional, i.e., not a regular Hebrew word or an abbreviation (see Note 4). The word בארי as such appears just twice in the Hebrew Bible, each time as a person’s name (Genesis 26:34; Hosea 1:1). In the present instance it may refer to a location and should be understood as “in Ari (ארי)” or as an actual place-name, i.e., “Bari.” Alternatively, it could be the singular possessive form of the word באר, i.e., “my elucidation” or “my clear rendering” (Deuteronomy 1:5; 27:8; Habakkuk 2:2).

In the absence of any known place of Jewish habitation in the 15^th century called Ari, the city of Bari in southern Italy, which had a flourishing Jewish community until the expulsion of the Jews from the kingdom of Naples in 1510-1511, would appear to be the better alternative (see Note 5). Assuming the other possibility, namely, that it is the singular possessive form of the word באר, the colophon would read “Behold my clear rendition, its transcription was completed …” It may even be that the copyist intended the word בארי to have a double meaning and to indicate both where the text was written and to point to the fine quality of his script.

The Russian State Library, Moscow, manuscript

The Hebrew version of al-Hassar’s treatise at the Russian State Library, Moscow, is titled “Abu Bakr’s Book of Fractions.” It is the fourth of six medieval Hebrew works on mathematics and related subjects collected in a codex (see Note 6). Its text is, however, somewhat different from that in the Vatican and Christ Church manuscripts: a number of the worked examples in those manuscripts have been omitted and others amended or added, the last third of the text is arranged differently, the mathematical notation is in places at variance with that in those two texts, and there are also numerous copyist errors throughout. The colophon (fol. 38r) gives the name of the copyist as Gad Ashtruck ben Yaacov (גד אשתרוק בן יעקב) and the year of its composition 5263 [1502/3]; there is no reference to the translator. This colophon is worded as a receipt for payments the copyist had received for lessons given to a person called Baruch and for the sale of a manuscript to him; the work is described as “… the book that I and al-Hassar, who is called Abu Bakr, wrote on Arithmetic …” At best, it appears to be an abridged redaction of Moses ibn Tibbon’s translation.

---

Note 1. Walī al-Dīn Abd al-Raḥmān ibn Muḥammad ibn Muḥammad ibn Abī Bakr Muḥammad ibn al-Ḥasan Ibn Khaldūn (1332-1406) was an Arab historian and historiographer who developed one of the earliest nonreligious philosophies of history in his Muqaddimah (Introduction). He is regarded as one of the founders of sociology, demography and economics.

Note 2. The missing text is that from fols. 98 to 127 in the Gotha manuscript, which corresponds in the Christ Church manuscript to the Hebrew text from the fourteenth line of the right-hand column of fol. 24v to the twenty first line of the right-hand column of fol. 31v and in the Vatican manuscript to that from the thirteenth line of fol. 56v to the second word in the fourth line from the bottom of fol. 76r.

Note 3. The correct order of the folios according to the flow of the text is: 1 to 9, 17, 19, 10 to 16, 18, 20 to 77.

Note 4. The same mark appears above anomalous words elsewhere in the manuscript – for example, in the phrase

(בחסרון הוו)

(the expression on the right is in Hebrew block letters), meaning “without the [Hebrew letter/conjunction] vav" – in the sub-sections numbered 61 to 69 in Part Two of the Christ Church Manuscript (fols. 16r to 17v). (Image used by permission of Christ Church College Library)

Note 5. The word בארי appears as the name of a location in the colophon of four other extant 15^th century Hebrew manuscripts: IMHM Film Nos. 14563 (1451), 13819 (1473), 14619 (1480 and 6405 (1487).

Note 6. The other five works are Abraham ibn Ezra’s Sefer ha-Mispar, Gersonides’ Maaseh Hoshev and three works composed by the copyist himself: a Treatise on Jewish Monetary Law and two short treatises on Arithmetic.

By the 10^th century, the knowledge preserved and developed in the Arabic Orient had begun making its way via the Maghreb to Spain, from where, after being translated into Latin, it would be taken up by European scholars. Chief amongst the translators of the Arabic scientific works to Latin was the Italian Gerard of Cremona (1114-1187) who had moved to Toledo in order to learn Arabic and access the city’s libraries of Arabic books. By contrast, the Jewish scholars in Andalusia and the Maghreb, who were proficient in both Hebrew and Arabic, would have had little recourse to translations: Maimonides’ famous Guide for the Perplexed, his Commentary on the Mishna, and his Responsa were all initially composed in Judeo–Arabic (Arabic written in Hebrew script). But their coreligionists in Provence and elsewhere in Europe would need Hebrew versions of these works.

Samuel ibn Tibbon (1150-1230) and his son, Moses ibn Tibbon, who was born in Marseille circa 1190 and died in 1283, were two of the most prolific Hebrew translators of their time. It was Samuel ibn Tibbon who first translated Maimonides’ Guide for the Perplexed into Hebrew as well as several chapters from his Commentary on the Mishna. His son Moses ibn Tibbon, a physician and author in his own right, is best known for his many Hebrew translations of Arabic works on philosophy, medicine, astronomy, and mathematics, of which the translation of Abu Bakr al-Hassar's Kitāb al-Bayān is an example.

The texts of the Vatican and Christ Church manuscripts are essentially identical and appear to have been copied from the same source. Not only do their versions of ibn Tibbon’s translation match, but both include the same appendix to al-Hassar’s treatise comprising a further nine examples and exercises. Whether these were added to the translation by Moses ibn Tibbon himself or by some later copyist is unknown but they must have been present in the source from which the texts of both manuscripts were copied. Apart from the inevitable copyist errors, such differences as there are between the Vatican and Christ Church manuscripts relate only to their scripts (Italian and Provencal/Sefardic, respectively), the layout of the script, and the annotations and corrections (marginalia) added by the respective copyists or later readers. In fact, there are numerous instances of the same error occurring in both versions. To cite just one case, the text of worked example 42 on the multiplication of fractions in both the Christ Church (fol. 12v) and Vatican (fol. 28v) manuscripts reads “… two thirds of five and five sixths by five sevenths of …”; however, the symbolic representation in both versions has “… two thirds of five and five sixths by six sevenths of ….” The versions of this example in the Arabic Gotha (Suter 1901, p. 26) and Schoenberg (fol. 44v) manuscripts both have “six sevenths,” to which the answer given in all four texts corresponds.

Indeed, other than the nine examples appended to the two Hebrew manuscripts and allowing for the differences that will inevitably arise when a text is translated into a different language, there is an almost one to one correspondence between ibn Tibbon’s Hebrew text and the Arabic texts in the Gotha and Schoenberg manuscripts. Every topic and worked example in the latter texts appears at the corresponding point in ibn Tibbon’s Hebrew translation. Even al-Hassar’s Arabic exaltations and invocations to Allah are matched by similar Hebrew praises and calls to God (Karpinski, p. 50). The one significant exception is in the opening lines of the work where al-Hassar explains what motivated him to write it. In Suter’s translation, the reason he gives is

that it was after I came to the realisation that the basis of [all] the sciences and fine literature, is the science of numbers, [coming of course] after Allah and the divine entities … (Suter 1901, p. 13).

The corresponding passage in the Hebrew translation reads:

Behold, God placed in numbers a hint of how to attain knowledge of His Oneness and of the order of His Creation, and by which to know every sealed and cryptic thing (fol. 1r, both Vatican and Christ Church manuscripts).

In both the Arabic and Hebrew versions, al-Hassar adds that he composed the work to be “a guide to beginners and a reminder to practitioners” and that

everything I have compiled, described and explained in this book, derives from the teachings of the older scholars, which I have logically clarified and expanded upon.

In their passage from east to west, the original Hindu (Indian) numerals evolved into a number of different forms. Our present European numerals are descended from the set of 11th century glyphs known as the Western Arabic or “Gobar” numerals (digits) that developed in the Maghreb and Andalusia (see Figure 4). The term “Gobar” derives from the Arabic ghubār, meaning a sand or dust board, the device on which the numerals that the Arabs developed from the original Hindu forms were first written. In his Hebrew translation of al-Hassar’s Kitāb al-Bayān, Moses ibn Tibbon translates the term as אבק = dust. The zero digit – ṣifr in Arabic and צפר in Hebrew – was a small circle.

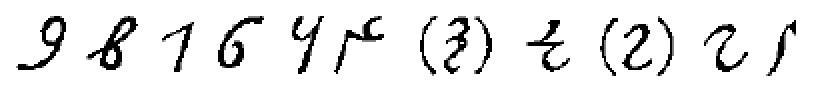Figure 4. Top row: The Gobar (Western Arabic) numerals (digits) from one to nine in the Gotha manuscript (in ascending order from right to left), as reproduced in Suter’s translation (1901, p. 15). Bottom row: The corresponding Eastern Arabic numerals on fol. 4r of the Schoenberg manuscript.

Whereas the 15^th century Italian copyist of the Vatican manuscript chose to employ a contemporary European form of the Gobar-based digits for the numbers in his text (Figure 5), the unnamed copyist of the Christ Church manuscript chose the first nine letters of the Hebrew alphabet, Aleph to Tet; not as gematria but as the digits of a positional decimal notation (Figure 6). Thus, writing the numbers from left to right in a Provencal/Sefardic Hebrew cursive script, he denotes the integer 543 by  

גדה

(the expression on the right is in modern Hebrew block letters), and he denotes the integer 583696 by

וטוגחה

as perhaps Moses ibn Tibbon did before him in his original translation (see Note). By contrast, in the decimal multiplication table on fol. 3v of the Christ Church manuscript, the copyist inscribed the numbers in the traditional Hebrew format from right to left (Figure 7).

Figure 5. The 15^th century European numerals used by the Italian copyist of the Vatican manuscript (fol. 2v). (Image used by permission of Vatican Library)

 

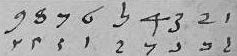	א     ב     ג     ד     ה     ו     ז     ח     ט

Figure 6. The schema in the margin of fol. 1v of the Christ Church manuscript showing the contemporary 15^th century European numerals and the Hebrew letters in cursive Provencal/Sefardic script used exclusively by the copyist for the digits from one to nine; the corresponding modern Hebrew block letters are shown below for reference. (Image used by permission of Christ Church College Library)

 

10          9          8          7          6          5         4         3         2         1
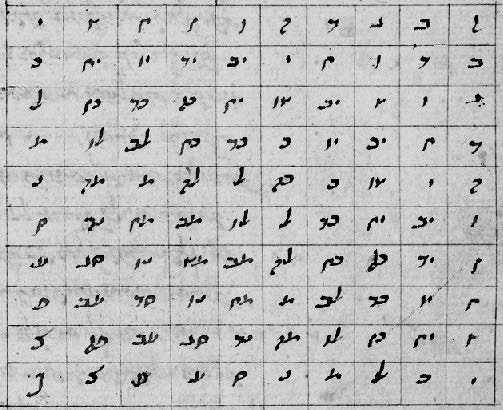	1 2 3 4 5 6 7 8 9 10

Figure 7. The decimal multiplication table on fol. 3v of the Christ Church manuscript in which the numbers are written in the traditional Hebrew format from right to left. The same table, though not as neatly drawn, appears in the Vatican manuscript (fol. 7v). (Image used by permission of Christ Church College Library)

---

Note. For a 15^th century use of the letters of the Greek alphabet as symbolic numerals, see Karl Menninger’s Number Words and Number Symbols, p. 274.

Al-Hassar’s Kitāb al-Bayān is a didactic text; it addresses the student directly and is written in a clear and user-friendly style. The worked examples all assume the student is working with a dust board. The methods of calculation require moving numbers around and rubbing out some as the work proceeds, a mode for which the dust board was well suited in the same way as are a black or white board, chalk or marker, and eraser (see Note 1). For these methods, finding the result was what mattered and not the intermediary steps. However, some algorithms suitable for paper and pen or pencil calculations appear in the appendices of both the Christ Church and Vatican manuscripts and therefore are possibly from as early as the 14th century. These algorithms are described in the last webpage of this article.

In his Introduction, al-Hassar states that he has arranged the work in two parts: the first on integers and the second on fractions. For our descriptive purposes, however, we will divide the text into three parts: Part One on integers; Part Two on fractions; Part Three on computations (see Note 2). By reason of its superior physical condition, the Christ Church manuscript will be our primary Hebrew source.

In Part One, integers and the operations associated with them are examined under ten chapter headings (see Note 3):

1. Numeration: On number scales (levels) and number names
2. Notation: The Gobar digits and their use in a positional decimal notation
3. Addition
4. Subtraction
5. Multiplication
6. Denomination
7. Division
8. Halving
9. Doubling
10. Extraction of Roots (see Note 4).

The chapter on Denomination is subtitled “Division of a Small Number by a Larger One,” and it is here that the horizontal bar notation first appears in the context of the naming and symbolic representation of the ratio of a smaller number to a larger one using Gobar numerals (integers). The chapter on Multiplication is followed by that on Denomination and not, as might have been expected, by that on Division, because the new notation would be required in the latter.

The chapter on Denomination opens with a review of the various classes of integers – prime or composite, odd or even – and of their divisibility and remainder rules. Division is treated as the repeated subtraction (השלכה) of the divisor (divider) from the dividend. Following this preamble, which al-Hassar states is an essential prerequisite to the subject at hand, the formal classical definition of the ratio of a smaller number to a larger one is presented:

The ratio of a smaller number to a larger one is the number of parts, one or more, that the former is of the latter.

This definition echoes that of Euclid: “Each number is either a part of a larger number [a unit fraction] or parts [a proper fraction] of it” (Elements VII.4). Al-Hassar obtains from it the following names (designations):

[Thus]…the ratio of one to three is called “a third” [one part of three] … that of one to four, “a fourth” [one part of four]; that of two to four, “two fourths" [two parts of four] … that of six to eight, “six eighths” [six parts of eight] … that of nine to ten, “nine tenths” [nine parts of ten].

Thus far, it is all quite straightforward and familiar. But al-Hassar now presents a different method for naming the ratios in those instances where the larger number can be factorized.

When it is said to you, “Name one part of fifteen.” Now you have already learned that fifteen is a composite number that arises from the multiplication of three by five; it follows, therefore, that three is one fifth of fifteen and five is one third of fifteen; thus, since one is a third of three, one part of fifteen [a fifteenth] is “one third of a fifth” \(\left[{\frac{1}{3}} \cdot{\frac{1}{5}}={\frac{1}{15}}\right]\).

Based on this example, al-Hassar proposes that seven parts of fifteen [seven fifteenths], might be symbolically represented by integers as follows: 

Write the factors \(3\) and \(5\) in a line, and put the \(7\) over the \(3\):

\[\genfrac{}{}{0pt}{}{7\quad \phantom{x}}{3\quad 5}\]

Now find a multiple of \(3\) which, when deducted from \(7,\) leaves a remainder less than \(3;\) the only possibility here is multiplier \(2\) and remainder \(1\) \([7-(2\times 3) = 1].\)

Delete the seven and replace it by the remainder, \(1;\) put the multiplier, \(2,\) over the \(5\):

\[\genfrac{}{}{0pt}{}{1\quad {2}}{3\quad 5}\]

Now draw a line between the two rows of numbers:  \[\frac{1\quad 2}{3\quad 5}\]

Al-Hassar reads this symbolic representation, from right to left, as “two fifths and a third of a fifth” (see Note 5):

\[\frac{1\quad 2}{3\quad 5}\implies\frac{2}{5}+\frac{1}{3\times 5}=\frac{7}{15}\]

He derives in a similar way a symbolic representation for eleven fifteenths: \[\frac{2\quad 3}{3\quad 5}\] He reads this notation, from right to left, as “three fifths and two thirds of a fifth”: \[\frac{2\quad 3}{3\quad 5}\implies\frac{3}{5}+\frac{2}{3\times 5}=\frac{11}{15}\] As we will see, whereas multi-digit integers are read from left to right in the direction of decreasing powers of ten, the symbolic representation of fractions is written and read from right to left.

In modern terminology, what al-Hassar contrived was a composite fraction notation in which a sequence of numerators and denominators are aligned, one above the other, with a horizontal line between them. Reading from right to left, each of the successive terms above the line is the numerator of the fraction whose denominator is the product of all the terms below and to the right of it. In the simplest case – two integers, \(a\) and \(b,\) above the line and two, \(c\) and \(d,\) below – this gives:

\[\frac{b\quad a}{d\quad c}\implies \frac{a}{c}+\frac{b}{dc}.\]

Employing this notation, a fifteenth is denoted in the Christ Church Library manuscript by

\[\frac{1\quad\phantom{0}}{3\quad 5}\implies \frac{0}{5}+\frac{1}{3\times 5}=\frac{1}{15},\]

seven fifteenths by

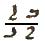\[\frac{1\quad 2}{3\quad 5}\implies \frac{2}{5}+\frac{1}{3\times 5}=\frac{7}{15},\]

and eleven fifteenths by

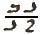\[\frac{2\quad 3}{3\quad 5}\implies \frac{3}{5}+\frac{2}{3\times 5}=\frac{11}{15}.\]

The copyist of the Christ Church manuscript chose to place the symbolic representations in the margins outside the running text, whereas they are embedded within the text in the Vatican manuscript (see Figure 8, below).

Figure 8. Folio 5r of the Christ Church manuscript. Reading from top to bottom, the three entries in the margin between the columns are:

\[\frac{1\quad 2}{3\quad 5}\implies \frac{7}{15},\quad\quad \frac{2\quad 3}{3\quad 5}\implies \frac{11}{15},\]

and

\[\frac{1\quad 0\quad 0}{2\quad 6\quad 8}\implies \frac{0}{8}+\frac{0}{6\times 8}+\frac{1}{2\times 6\times 8}=\frac{1}{96}\]

As shown by this last example, the notation can be expanded to more terms via formulas of the form \[\frac{c\quad b\quad a}{f\quad e\quad d}\implies\frac{a}{d}+\frac{b}{ed}+\frac{c}{fed}\] and so on. (Image used by permission of Christ Church College Library)

Al-Hassar's novel composite fraction notation was later taken up by Fibonacci (c. 1175-1250) in Chapter 5 of his Liber Abaci (1202). This notation also appears in a work by the Maghreb mathematician ibn al Yasamin (d. 1204), Talqih al-afkar bi rushum huruf al-ghubar, or Fertilization of Thoughts with the Help of Dust Letters (Lamrabet). However, it would take another three centuries for fraction notation to evolve into the simpler notation now commonly used for all vulgar (common) fractions.

---

Note 1. According to A. S. Saidan, “Hindu arithmetic entered Islam with the dust board (takht) as an intrinsic tool of it, writing and rubbing out being made by the fingers or with a stylus” (p. 351). There is no clear evidence, however, for use of the dust board in India.

Note 2. In his translation of the Gotha manuscript, Suter (1901) divides the treatise into seven Chapters (Kapiteln): our Parts 1 and 2 correspond to his Chapters 1 and 2; our Part 3 encompasses his Chapters 3 to 7. The Gotha manuscript does not have the appendices found in the two Hebrew manuscripts.

Note 3. Folios 1r to 7r in the Christ Church manuscript and folios 1r to 9v, 17r to 17v and 10r to 14r (in that order), in the Vatican manuscript

Note 4. This chapter only deals with the roots of “square” numbers, e.g. 625 (sq. rt. = 25) and 583696 (sq. rt. = 764). Extracting the square roots of integers and fractions in general is the final topic in al-Hassar’s treatise; it appears in folios 30r to 31v in the Christ Church codex, folios 69v to 73r in the Vatican manuscript, and pages 37 to 39 (Siebentes Kapitel) in Suter’s translation of the Gotha manuscript.

Note 5. Henceforth, the symbol \(\implies\) will indicate a replacement of al-Hassar's representation of arithmetic operations by modern notation.

Al-Hassar's chapter, "Denomination," considered on the preceding page, is subtitled “Division of a Small Number by a Larger One.” The reverse operation, “Division of a Large Number by a Smaller One,” is taken up in the chapter headed “Division” (the seventh of the ten chapters in Part One of the Kitāb al-Bayān) and it is here that al-Hassar first demonstrates an application of his new notation. The context is how to deal systematically with the remainder when the dividend is not an integer multiple of the divisor. The example he gives (at fol. 5v in the Christ Church manuscript and fol. 11r in the Vatican manuscript) is the division of ninety-eight thousand seven hundred and forty-six (98746) by thirty-six (36).

The compound number thirty-six has the factors four and nine, so first divide the ninety-eight thousand seven hundred and forty-six by four. This gives twenty-four thousand six hundred and eighty-six with two remaining over the four. Now divide the twenty-four thousand six hundred and eighty-six by nine. This gives two thousand seven hundred and forty-two with eight remaining over the nine \([98746\div 4 = 24686\,{\rm{R}}\,2\) and \(24686\div 9 = 2742\,{\rm{R}}\,8].\)

Al-Hassar now shows how the two remainders, the two over four from the first division and the eight over nine from the second, can be combined using his new notation. Mixed numbers comprising an integer and a simple fraction are written with the integer to the right of the fraction.

Draw a horizontal line and write the nine and the four beneath it with the nine on the right and the four on the left. Now place the eight over the nine and the two over the four. Placing the integer [part of the quotient] to its right gives the final answer, two thousand seven hundred and forty-two and eight ninths and two fourths of a ninth \[\frac{2\quad 8}{4\quad 9}\,2742\implies 2742+\frac{8}{9}+\frac{2}{4\times 9}=2742\,\frac{17}{18}.\]

(See Note.) In this neat way, the remainders from any sequence of divisions by the factors of a compound divisor can be combined using al-Hassar’s new notation. He now goes on to show how, having obtained this expression, the answer can be checked using the technique of “casting out sevens.”

The answer will be correct if dividing both the dividend and the quotient by seven leaves the same remainder. Dividing the dividend by seven gives a remainder of four \([98746\div 7 = 14106\,{\rm{R}}\,4].\)

Dividing the integer part of the quotient by seven leaves a remainder of five \([2742\div 7 = 391\,{\rm{R}}\,5].\)

Dividing the nine below the line in the fractional part of the quotient by seven leaves a remainder of two and multiplying this by the remainder five from the integer part gives ten \([9\div 7 = 1\,{\rm{R}}\,2\) and \(2\times 5=10].\)

Dividing this ten by seven leaves a remainder of three. To this add the remainder of one that is left from dividing the eight that is above the nine by seven; this gives four \([10\div 7 = 1\,{\rm{R}}\,3,\,\,8\div 7 = 1\,{\rm{R}}\,1,\) and \(1 + 3=4].\)

Multiply this four by the other number below the line, namely, the four; this gives sixteen. Dividing the sixteen by seven leaves a remainder of two which when added to the two above the four in the expression gives four \([4\times 4=16,\,\,16\div 7 = 2\,{\rm{R}}\,2,\) and \(2+2=4].\)

Thus, when they are divided by seven, the dividend and quotient leave the same remainder, i.e., four, which bears out the correctness of the result.

---

Note. Again, the symbol \(\implies\) indicates replacement of al-Hassar's representation of arithmetic operations by modern notation.

The focus of Part Two of al-Hassar's Kitāb al-Bayān is on the multiplication of simple, mixed, complex and compound fractions (see Note 1). It comprises seventy-two sub-sections or headings (שערים), in the first of which the new notation by which a horizontal bar (vinculum) separates the numerator and denominator of a fraction is formally presented along with its basic usages and applications (see Note 2).

The first fraction is a half, followed by a third, a fourth, a fifth … and to depict a half, write a two and draw a line above it, and over the line write a one thus \(\frac{1}{2}\) … and for a third, a three and a one over it thus \(\frac{1}{3}\) … and for two thirds, write a two in place of the one \(\frac{2}{3},\) and so on.

All simple fractions whose denominators are ten or less, or a prime number greater than ten, are represented in this way; for example, a quarter, five sixths, an eleventh, two thirteenths, six nineteenths: \[ \frac{1}{4},\,\,\frac{5}{6},\,\,\frac{1}{11},\,\,\frac{2}{13},\,\,\frac{6}{19},\] respectively, in modern Arabic numerals. The Hindus (Indians) wrote the denominator under the numerator but without the horizontal bar. The horizontal bar first appeared in Europe in Fibonacci’s Liber Abaci (1202), an innovation that he took from Arab sources.

Al-Hassar next moves on to the symbolic representation of those simple fractions whose denominators are greater than ten and can be factorised. These are designated "by two names" – i.e., as a fraction of a fraction. For example: a twelfth (a half of a sixth),

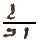\[\frac{1\quad {\phantom x}}{2\quad 6}\implies \frac{0}{6}+\frac{1}{2\times 6}=\frac{1}{12}\]

(see Note 3); a twenty-eighth (a quarter of a seventh),

\[\frac{1\quad {\phantom x}}{4\quad 7}\implies \frac{0}{7}+\frac{1}{4\times 7}=\frac{1}{28};\]

ten fifteenths or two thirds (three fifths and a third of a fifth),

\[\frac{1\quad {3}}{3\quad 5}\implies \frac{3}{5}+\frac{1}{3\times 5}=\frac{10}{15}=\frac{2}{3};\]

twenty-one twenty-fourths or seven eighths (five sixths and a quarter of a sixth),

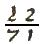\[\frac{1\quad {5}}{4\quad 6}\implies \frac{5}{6}+\frac{1}{4\times 6}=\frac{21}{24}=\frac{7}{8};\]

and forty-seven one hundred and forty-threes (four thirteenths and three elevenths of a thirteenth),

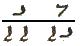\[\frac{3\,\,\,\,\,\,\, {4}}{11\,\,\,\,\, 13}\implies \frac{4}{13}+\frac{3}{11\times 13}=\frac{47}{143}.\]

Al-Hassar’s representation is similar for fractions designated by three or more names; i.e., a fraction of a fraction of a fraction, or a fraction of a fraction of a fraction of a fraction, and so on. For example,

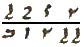\[\frac{1\quad 5\quad 7\,\,\,\, \phantom{1} 9}{2\quad 6\quad 9\,\,\,\,\, {11}} \]

reads nine elevenths and seven ninths of an eleventh and five sixths of a ninth of an eleventh and half of a sixth of a ninth of an eleventh; i.e., ninety-seven parts of one hundred and eight: \[\frac{1\quad {5}\quad {7}\quad {9}}{2\quad 6\quad {9}\quad {11}}\implies \frac{9}{11}+\frac{7}{9\times 11}+\frac{5}{6\times 9\times 11}+\frac{1}{2\times 6\times 9\times 11}=\frac{1067}{1188}=\frac{97}{108}.\]

Turning to other applications of his new notation, al-Hassar enjoins that the terms in a row of unrelated simple fractions – for example, three quarters, four fifths, five sixths, six sevenths and ten elevenths – should be clearly separated from one another: this is realised in the Christ Church manuscript (fol. 7v) by means of vertical strokes,

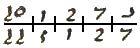and in the Vatican manuscript by blank spaces (see Note 4), \[\frac{10}{11}\,\,\frac{6}{7}\,\,\frac{5}{6}\,\,\frac{4}{5}\,\,\frac{3}{4}.\] Numbers comprising an integer and a simple or composite fraction (mixed fractions) are denoted with the integer to the right of the fraction. (Fibonacci followed this Arab practice of placing the fraction to the left of the integer.) For example (see Note 5), eight and two sixths is written with \(\frac{2}{6}\) to the left of \(8,\)

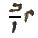\[\frac{2}{6}\,8\implies 8\,\frac{1}{3},\]

and one and a seventh and a third of a seventh is written with \(1\) to the right of the fraction,

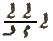\[\frac{1\quad {1}}{3\quad 7}\,1\implies 1+\frac{1}{7}+\frac{1}{3}\cdot \frac{1}{7}=1\,\frac{4}{21}.\]

Conversely, when the fraction (simple or composite) is to the right of a number, it indicates taking that fraction of the number. For example, three fourths of a fifth of eight (three twentieths of eight) is written as follows:

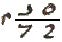\[8\,\frac{3\quad {0}}{4\quad 5}\implies \left(\frac{0}{5}+\frac{3}{4\times 5}\right)\times 8 ={\frac{3}{20}}\times 8=\frac{24}{20}=\frac{6}{5}.\]

Standardised notations such as the now familiar arithmetical signs (+, –, ×, ÷, etc.) only came into use in the late sixteenth and early seventeenth centuries with the spread of printed mathematical books. In their absence, arithmetical operations were often indicated by a juxtaposition. Thus, for example, the simple addition of two fractions is represented in al-Hassar’s treatise by placing their symbolic representations side by side:

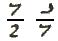\[\implies\frac{4}{5}+\frac{3}{4}.\]

A notation could, however, have more than just one usage. For example, the constituent fractions represented by the sequence of numerators and denominators in the composite fraction notation are linked together by the conjunction "and" (= plus). In this way, the composite fraction at left (below) is read (from right to left) as three fourths and four fifths of a fourth and five sixths of a fifth of a fourth: \[\frac{5\quad 4\quad 3}{6\quad 5\quad 4}\implies \frac{3}{4}+\frac{4}{4\times 5}+\frac{5}{4\times 5\times 6}.\]

Al-Hassar now adds that this notation can have an alternate usage; namely, that it can also be read as a sequence of simple fractions without the conjunction ‘and’ (+), such that each fraction is that part of the following fractions. Taking the above example, this gives (reading from right to left) three fourths of four fifths of five sixths; i.e., in modern notation, the product \[\frac{3}{4}\times \frac{4}{5}\times \frac{5}{6}.\] The Andalusian mathematician, al-Qalasadi (1412-1486), differentiated between the two usages by inserting a vertical line between the individual fractions in the latter (Suter 1901, p. 27): \[\frac{5\,\vert\, 4\,\vert\, 3}{6\,\vert\, 5\,\vert\, 4}.\] This latter usage is exercised in al-Hassar's Kitāb al-Bayān in sub-sections 60 to 69 of Part One: Christ Church manuscript fol. 16r and Vatican fols. 36v to 42v.

---

Note 1. This section occupies fols. 7r–18r in the Christ Church manuscript, 14r–42r in the Vatican manuscript, and pp. 23–28 in Suter’s translation.

Note 2. Sub-section 1 appears on fols. 7r and 7v in the Christ Church manuscript and fols. 14r and 14v in the Vatican manuscript.

Note 3. Once again, the symbol \(\implies\) indicates replacement of al-Hassar's representation of arithmetic operations by modern notation. All images of handwritten Hebrew text are used by permission of Christ Church College Library.

Note 4. Vatican manuscript, fol. 14v. There is some confusion here. Although the text in the Vatican manuscript has “…four fifths…,” the copyist entered a 2 under the 4 in the symbolic representation and not a 5. Furthermore, the words “six sevenths” do not appear in the text though the fraction is included in the symbolic representation. And to compound it all, neither the words nor the fraction appear in the Schoenberg manuscript (fol. 25v), which also has spaces between the terms.

Note 5. The two examples that follow occur in sub-sections 2 and 4, respectively: fol. 7v in the Christ Church manuscript and fols. 15r and 15v in the Vatican manuscript.

In each of the remaining seventy-one sections of Part Two of al-Hassar's Kitāb al-Bayān, a different calculation involving the multiplication of a fraction (or fractions) is exemplified: an integer by a simple fraction; a composite fraction by a mixed fraction; a composite fraction by another composite fraction; a fraction of a mixed fraction (simple or composite) by an integer, a simple fraction, a composite fraction or another mixed fraction (simple or composite); and so on. Each of these “how to” worked examples starts with the words “When it is said to you (כשיאמר לך) …” In a number of instances, al-Hassar shows how to check the answer arrived at by the technique of “casting out” and some of the more advanced or complex examples are also followed by a scholium (פרק). Suter remarks: “These sections are the richest of all known examples of fractions in Arabic arithmetic books, so extensive that it appears tiring, unwieldy and confusing for the practitioner” (1901, p. 24). Indeed, at times the work reads almost like a manual or recipe book. Not surprisingly, there are numerous copyist errors in both Hebrew versions, many of which were later noticed and corrected in the margins.

The following are a representative sample of these worked examples.

Example 1. "On the multiplication of a fraction by an integer." (See Note 1.)

When it is said to you, multiply five sixths by ten. Place the five sixths on one row and the ten on the row below, in this way:

\[\genfrac{}{}{0pt}{}{\frac{5}{6}}{10}\]

Multiply the five above the six by the ten, \(5\times 10=50,\) and divide the result by six, \(50\div 6 = 8\frac{2}{6}.\) The answer is eight and two sixths.

Example 2. "On the multiplication of a composite fraction by an integer." (See Note 2.) 

When it is said to you, multiply a fifth and a half of a fifth by twelve. Place the fifth and a half of a fifth on one row and the twelve on the row below, in this way: 

\[\genfrac{}{}{0pt}{}{\frac{1\,\,1}{2\,\,5}}{12}\]

Multiply the one above the five by two, \(1\times 2 = 2,\) and add to this the one that is above the two: \(1+2=3.\) Multiply the three by twelve, \(3\times 12=36,\) and divide the result by the denominators; i.e., by two, \(36\div 2=18,\) followed by five, \(18\div 5 = 3\frac{3}{5}.\) The answer is three and three fifths.

Example 3. "On the multiplication of a simple fraction by another simple fraction." (See Note 3.)

When it is said to you, multiply seven eighths by nine tenths. Place the seven eighths on one row and the nine tenths on a row below, in this way:

\[\genfrac{}{}{0pt}{}{\frac{7}{8}}{\frac{9}{10}}\]

Multiply the seven above the eight by the nine above the ten, \(7\times 9 = 63,\) and divide the result by the denominators; i.e., by eight, \(63\div 8=7\frac{7}{8},\) followed by ten, \(7\frac{7}{8}\div 10 = \frac{7}{10}+\frac{7/8}{10}.\) The answer is seven tenths and seven eighths of a tenth:

\[\frac{7\,\,\quad {7}}{8\quad {10}}\implies \frac{7}{10}+\frac{7}{8\times {10}}=\frac{63}{80}.\]

Example 4. "On the multiplication of a composite fraction by a simple fraction." (See Note 4.)

When it is said to you, multiply six sevenths and a third of a seventh by eight ninths. Place the six sevenths and a third of a seventh on one row and the eight ninths on a row below, in this way.

\[\genfrac{}{}{0pt}{}{\frac{1\,\,\,6}{3\,\,\,7}}{\frac{8}{9}}\]

Multiply the six above the seven in the upper multiplicand by the three below the line, \(6\times 3=18,\) and add the product to the one above the line making nineteen. Multiply this by the eight in the lower multiplicand, \(19\times 8=152.\) Divide the one hundred and fifty-two by the denominators of the two multiplicands; i.e., by three, \(152\div 3=50\frac{2}{3},\) followed by seven, \(50\frac{2}{3}\div 7 = 7+\frac{1\, 2/3}{7},\) followed by nine: \(\left(7+\frac{1\,2/3}{7}\right)\div 9=\frac{7}{9}+\frac{1/7}{9}+\frac{\frac{2/3}{7}}{9}.\) This gives seven ninths and a seventh of a ninth and two-thirds of a seventh of a ninth:

$$\frac{2\,\,\,1\,\,\,7}{3\,\,\,7\,\,\,9}$$

Example 5. "On the multiplication of the sum of two fractions by an integer." (See Note 5.)

When it is said to you, multiply the sum of three quarters and four fifths by fifteen. Place the three quarters and four fifths in a row with the fifteen below them, in this form:

$$\genfrac{}{}{0pt}{}{\frac{4}{5}\,\,\,\frac{3}{4}}{15}$$

Starting with the row of fractions, multiply the three (the numerator) that is over the four by the five (the denominator) under the four \(\left(3\times 5=15\right)\) and the four (the numerator) that is over the five by the four (the denominator) under the three \(\left(4\times 4=16\right).\) Adding the two products gives thirty-one: \(15+16=31.\)

Multiplying thirty-one by fifteen gives four hundred and sixty-five: \(31\times 15=465.\) Dividing this by the denominators of the two fractions, four followed by five, gives twenty-three and one fifth and a quarter of a fifth: \(465\div 4=116\frac{1}{4}\) then  \[116\frac{1}{4}\div 5 = 23\frac{1}{5}+\frac{1/4}{5}\impliedby\frac{1\,\,\,1}{4\,\,\,5}\,23.\]

Example 6. "On the multiplication of a fraction of one integer by a different fraction of another integer." (See Note 6.)

When it is said to you, multiply five sixths and half a sixth of eight by eight ninths and a fifth of a ninth of twelve:

		\[8\,\frac{1\,\,\,5}{2\,\,\,6}\]
		\[12\,\frac{1\,\,\,8}{5\,\,\,9}\]

Taking the top row first, multiply the five over the six by the two in the denominator, \(5\times 2 = 10\); add the product to the one above the line, \(10+ 1 = 11\); and multiply the sum by eight, \(11\times 8 = 88.\)

Moving to the second row, multiply the eight over the nine by the five in the denominator, \(8\times 5 = 40\); add the product to the one above the line, \(40+ 1 = 41\); and multiply the sum by twelve, \(41\times 12 = 492.\)

Multiply the eighty-eight by the four hundred and ninety-two, \(88\times 492 = 43296.\) Divide the forty-three thousand two hundred and ninety-six by the denominators of the two composite fractions, two, five, six, and nine, as follows: \[43296\div 2 = 21648\frac{0}{2}=21648,\quad 21648\div 5 = 4329\frac{3}{5},\] \[4329\frac{3}{5}\div 6 = 721\frac{3}{6}+\frac{3/5}{6},\] \[\left(721\frac{3}{6}+\frac{3/5}{6}\right)\div 9 = 80\frac{1}{9}+\frac{3/6}{9}+{\frac{\frac{3/5}{6}}{9}}.\]

This gives eighty and a ninth and three sixths of a ninth and three fifths of a sixth of a ninth and zero halves of a fifth of a sixth of a ninth [we did not carry the \(0\) through in the work above]:

\[\frac{0\,\,\,3\,\,\,3\,\,\,1}{2\,\,\,5\,\,\,6\,\,\,9}\,80\implies80\frac{48}{270}=80\frac{8}{45}.\]

Dividing by the denominators in the reverse order – nine, six, five and two – produces a different but equivalent composite fraction; i.e., one with the same value: \[\frac{6\,\,\,4\,\,\,1\,\,\,0}{9\,\,\,6\,\,\,5\,\,\,2}\,80\implies80\frac{96}{540}=80\frac{8}{45}.\]

Because of the different ways in which a juxtaposition could be understood – addition in the case of two fractions or taking that fraction of a number when the fraction (simple or composite) is to the right of the number – ambiguities could arise, especially where complex calculations were concerned. Example 7 illustrates this.

Example 7. "On the multiplication of a fraction and an integer and two simple fractions and a whole number and a fraction by a similar expression." (See Note 7.)

When it is said to you, multiply three fourths of five and a half and five sixths of three and two fifths by two thirds of four and a seventh and three eighths of two and three elevenths, write down the question in this form [reading from right to left]:

	\[\frac{2}{5}\,3\,\frac{5}{6}\,\frac{1}{2}\,5\,\frac{3}{4}\]
	\[\frac{3}{11}\,2\,\frac{3}{8}\,\frac{1}{7}\,4\,\frac{2}{3}\]

The answer is \[\frac{0\,\,\,6\,\,\,5\,\,\,5\,\,\,5\,\,\,1}{10\,\,7\,\,\,8\,\,\,8\,\,\,9\,\,11}\,25\implies25\frac{6593}{44352}=25.1486516955 \dots.\]

To arrive at this result, al-Hassar had read each of the symbolic representations as the sum of two parts, each a fraction of a mixed fraction. In modern notation, this gives:

\[{\left({\frac{3}{4}\times5\frac{1}{2}+\frac{5}{6}\times3\frac{2}{5}}\right)}\times{\left({\frac{2}{3}\times4\frac{1}{7}+\frac{3}{8}\times2\frac{3}{11}}\right)}=25.1486516955 \dots.\]

However, because of the different ways in which a juxtaposition can be understood other readings were also possible. Al-Hassar was clearly aware of this and so he continues:

This question can be read in different ways. For example, take the five sixths from the second part of [the expression in the top row] and join it to the first part, whereupon the first part becomes taking a fraction of an integer and two fractions. [See Note 8.] This gives: \[\frac{2}{5}\,3\,\frac{5}{6}\,\frac{1}{2}\,5\,\frac{3}{4}\implies\frac{3}{4}\left(5+\frac{1}{2}+\frac{5}{6}\right)+3\frac{2}{5}.\] Furthermore, the expression does not have to be read as the sum of two parts; it could just as well be read as the sum of three, four, five or even more parts.

In the scholium that follows, al-Hassar provides other examples of how this expression could be read; for instance, in two parts: \[\frac{2}{5}\,3\,\frac{5}{6}\,\frac{1}{2}\,5\,\frac{3}{4}\implies\frac{3}{4}\times 5+\left(\frac{1}{2}+\frac{5}{6}\right)\times3\frac{2}{5},\] or in three parts: \[\frac{2}{5}\,3\,\frac{5}{6}\,\frac{1}{2}\,5\,\frac{3}{4}\implies\frac{3}{4}+\left(5\frac{1}{2}+\frac{5}{6}\right)+3\frac{2}{5}\] or \[\frac{2}{5}\,3\,\frac{5}{6}\,\frac{1}{2}\,5\,\frac{3}{4}\implies\frac{3}{4}\times 5+\frac{1}{2}\times\frac{5}{6}+3\frac{2}{5}.\]

Arithmetical texts were all handwritten at the time which would only have added even more ambiguities to those already inherent in the absence of standardised signs and the reliance on juxtapositions. In al-Uqlīdisī’s treatise, groups of numbers are in some instances surrounded by lines apparently to separate them from the writing around them, but this is not systematically adhered to. Addition and multiplication are likewise indicated in places by the insertion of three dots, ∴ (the modern handwritten “therefore sign”), between the numbers, though, as often as not, they too are omitted (Saidan, p. 423).

---

Note 1. Sub-section 2: fol. 7v in the Christ Church manuscript and fol. 14v in the Vatican manuscript. All images of handwritten Hebrew text are used by permission of Christ Church College Library. Throughout this webpage, the symbol \(\implies\) will indicate replacement by modern notation of al-Hassar's representation of arithmetic operations. Furthermore, we will use the modern symbols, \(+,\times,\div,\) and \(=,\) along with parentheses, to clarify our translations.

Note 2. Sub-section 3: fol. 7v in the Christ Church manuscript and fol. 15r in the Vatican manuscript. There is a copyist error in the wording of the example in the Christ Church manuscript. It reads “multiply two fifths …” and not “a fifth” whereas the answer given requires that it be the latter. The symbolic representation of the calculation is, however, correct as is the text in the Vatican manuscript.

Note 3. Sub-section 28: fol. 11r in the Christ Church manuscript and fol. 24v in the Vatican manuscript.

Note 4. Sub-section 29: fol. 11r in the Christ Church manuscript and fol. 25r in the Vatican manuscript.

Note 5. Sub-section 5: fol. 8r in the Christ Church manuscript and fol. 15v in the Vatican manuscript. A copyist error in the Christ Church manuscript gives the final answer in this example as “twenty-three and two fifths and a quarter of a fifth.”

Note 6. Sub-section 39: fol. 12v in the Christ Church manuscript and fol. 28r in the Vatican manuscript.

Note 7. Sub-section 58: fol. 15v in the Christ Church manuscript and fol. 35r in the Vatican manuscript.

Note 8. Al-Hassar adds that a calculation of this type has already been exemplified in sub-section forty-four.

In the last two sub-sections in Part Two, nos. 71 and 72 (see Note 1), al-Hassar introduces a novel way of representing the subtraction of a fraction, which may be one of the earliest instances of an arithmetical sign. His innovation was to employ the Arabic word إلا (illâ = except for) as what we would now term a minus sign in the representation of multiplications involving fractions (see Note 2); in the Hebrew versions, the Arabic word illâ is translated as אלא. Its usage was not, however, standardised and its import was modulated by how the various juxtapositions were read. For example, in sub-section 71:

On the Multiplication of a Fraction with a Stipulation (בתנאי). [See Note 3.] When it is said to you, multiply three fourths lacking (חסר) a sixth by four fifths except for (אלא) a third, write down the question in this form:

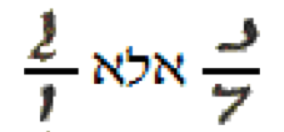		\[\frac{1}{6}\,\,ill\hat{a}\,\,\frac{3}{4}\]
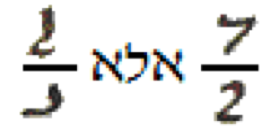		\[\frac{1}{3}\,\,ill\hat{a}\,\,\frac{4}{5}\]

This can be read in two different ways (see Note 4):

(i.) Take three fourths minus a sixth and multiply it by four fifths minus a third: \[\left({\frac{3}{4}-\frac{1}{6}}\right)\times\left({\frac{4}{5}-\frac{1}{3}}\right)=\frac{49}{180}.\]

Al-Hassar gives the result for this reading as:

\[\frac{3\,\,\,3\,\,\,2}{5\,\,\,8\,\,\,9}\implies\frac{2}{9}+\frac{3}{8\times 9}+\frac{3}{5\times 8\times 9}=\frac{98}{360}=\frac{49}{180}.\]

(ii.) Take three fourths minus a sixth of three fourths and multiply the result by four fifths minus a third of four fifths:

\[\left({\frac{3}{4}-\frac{1}{6}\cdot\frac{3}{4}}\right)\times\left({\frac{4}{5}-\frac{1}{3}\cdot\frac{4}{5}}\right) =\frac{15}{24}\times\frac{8}{5}=\frac{8}{24}=\frac{1}{3}.\]

The second of the sub-sections, no. 72, is headed, “On the Multiplication of a Fraction of a Number with a Stipulation (בתנאי).” The worked example reads:

When it is said to you, multiply three fourths of five except for (אלא) a sixth by four fifths of three except for (אלא) a fourth, write down the question in this form:

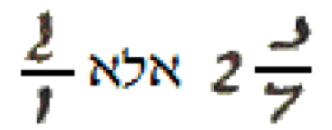		\[\frac{1}{6}\,\,ill\hat{a}\,\,5\frac{3}{4}\]
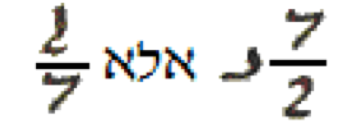		\[\frac{1}{4}\,\,ill\hat{a}\,\,3\frac{4}{5}\]

This can be read in three different ways:

(i.) Take three fourths of five minus a sixth of five and multiply it by four fifths of three minus a fourth of three:

\[\left({\frac{3}{4}\cdot 5-\frac{1}{6}\cdot 5}\right)\times\left({\frac{4}{5}\cdot 3-\frac{1}{4}\cdot 3}\right)=4\frac{65}{80}.\]

Al-Hassar gives the result for this reading as:

\[\frac{0\,\,\,1\,\,\,8}{6\,\,\,8\,\,10}\,4\implies 4\frac{8}{10}+\frac{1}{8\times 10}=4\frac{65}{80}.\]

(ii.) Take three fourths of five minus a sixth of three fourths of five and multiply it by four fifths of three minus a fourth of four fifths of three:

\[\left({\frac{3}{4}\cdot 5-\frac{1}{6}\cdot \frac{3}{4}\cdot 5}\right)\times\left({\frac{4}{5}\cdot 3-\frac{1}{4}\cdot \frac{4}{5}\cdot 3}\right)=5\frac{5}{8}.\]

(iii.) Take three fourths of five minus a sixth and multiply it by four fifths of three minus a fourth:

\[\left({\frac{3}{4}\cdot 5-\frac{1}{6}}\right)\times\left({\frac{4}{5}\cdot 3-\frac{1}{4}}\right)=7\frac{169}{240}.\]

Al-Hassar concludes: “What we have said about the multiplication of fractions should suffice for any person who studies it attentively …. And may God, the guide, show us the way to what is right.”

---

Note 1. Fol. 18r in the Christ Church manuscript and fols. 41v-42v in the Vatican manuscript.

Note 2. The same Arabic word was used in a similar way some two hundred years later in the Miftāḥ al Ḥ̣isāb, written by the Persian astronomer and mathematician, Jamshīd al-Kāshī (c. 1380–1429) (Saidan, p. 424).

Note 3. “… mit Ausschliessung (istitnâ)” – i.e., “an exception” – in Suter’s translation (1901, p. 28).

Note 4. All images of handwritten Hebrew text are used by permission of Christ Church College Library. The symbol \(\implies\) will continue to indicate replacement by modern notation of al-Hassar's representation of arithmetic operations. Furthermore, we will continue to use the modern symbols, \(-,\times,\cdot,\) and \(=,\) along with parentheses, to clarify our translations.

Part Three of al-Hassar's Kitāb al-Bayān encompasses a variety of operations and computations involving fractions and combinations of integers and fractions. These include the transformation, addition and subtraction of fractions; summations of numerical series; examples of useful commercial and monetary calculations; the famous wheat and chessboard problem; algebraic type computations in which the value of an unknown quantity (šai in Arabic, translated as דבר in Hebrew and meaning ‘a thing’) is sought; and some novel methods for extracting exact and/or approximate square roots of integers and fractional numbers. Here, too, many of the worked examples are accompanied by a scholium (פרק).

Taking the designations in al-Hassar’s treatise as our guide, the text of Part Three can be viewed as comprising five chapters, each composed of headings and sub-headings: in each sub-heading, a specific type of calculation or problem is first delineated and then exemplified by one or more worked examples or solutions. For the source of the chapter or sub-section in the Christ Church, Vatican, and/or Gotha (as translated by Suter in 1901) manuscripts, see the table in Note 1.

3.1       Transforming Fractions (שער פריטה).

3.1.1.   Changing a Fraction’s Denominator (five sub-headings).

3.2       Addition.

3.2.1.   The Addition of Fractions (nine sub-headings).

3.2.2.   Monetary Calculations (five sub-headings).

3.2.3.   The Summation of Numerical Series (ten sub-headings).

3.2.4.   The Article on Doubling (המאמר בכפול).

If a chessboard were to have grains of wheat placed upon each square such that one was placed on the first square, two on the second, four on the third, and so on; how many grains of wheat would there be on the board at the finish?

3.3       Subtraction (שער ההשלכה).

3.3.1.   The Subtraction of Fractions (four sub-headings).

3.3.2.   Monetary Calculations (seven sub-headings).

3.3.3.   The Reed and Fish Problems.

(i) A reed, standing in the mud by a river bank, has a third of its length in the mud, a quarter in the water and 10 spans (units of length) showing above the water. How long is the reed? [Ans. 24 spans]

(ii) A reed, standing in the mud by a river bank, has a third of its length and two spans in the mud, a quarter of its length and three spans in the water and 10 spans (units of length) showing above the water. How long is the reed? [Ans. 36 spans]

(iii) If a fish’s head is a third of its weight, the tail a quarter and its middle weighs 10 pounds, how heavy is the fish? [Ans. 24 pounds]

3.4       Division (שער החלוקה).

3.4.1.   Division of a Smaller Number by a Larger One (twenty-two sub-headings).

3.4.2.   Division of a Larger Number by a Smaller One (twenty-six sub-headings).

3.4.3.   The Augmenting (חיתום) of Fractions.

Know that this section is of great assistance in the whole of arithmetic and especially in algebra (Hebrew: חיתום; Arabic: el-ğebr; see Note 2).

If it is said to you, by how much must a third be augmented in order to become one? In other words, by what number must a third be multiplied to make one? Answer: By one divided by a third.

3.4.4.   The Reduction (ירידה) of a Fraction.

Know that Reduction (Hebrew: ירידה; Arabic: el-ḥatt) is the reverse of Augmenting.

If it is said to you, by how much must one be reduced in order to become a half? In other words, by what must one be multiplied to make a half? Answer: By a half divided by one.

3.5       Extracting the Roots of Integers and Fractions.

3.5.1.   Finding the Roots of Integers and Fractions that have Exact (Rational) Square Roots.

3.5.2.   Finding the Approximate Roots of Integers and Fractions that do not have Exact (Rational) Square Roots.

A full and detailed description of all the computations is far beyond the remit of this article. Suffice to say, that although al-Hassar employs his new composite notation throughout, the underlying mathematics is not new. Indeed, the topics and the order in which they appear are little changed from that in earlier Arabic arithmetical texts.

The last worked example before the colophons on fol. 31v of the Christ Church codex is “Find the square root of three sevenths.” Al-Hassar's instructions, using modern notation, are as follows:

Multiply \(\frac{3}{7}\) by the square of its denominator: \(49\cdot\frac{3}{7}=21;\) then take the square root of \(21\) which is approximately \(4\frac{3}{5}\) and divide it by the square root of \(49:\,\,4\frac{3}{5}\div 7=\frac{23}{35}.\) The result is a fairly close approximation to the square root of \(\frac{3}{7}.\) (See Note 3.)

The method used here is typical of those al-Hassar employs to find approximate values for irrational square roots. It is also the last item in Suter’s German translation of the Gotha manuscript and is, presumably, where both al-Hassar’s original treatise and Moses ibn Tibbon’s Hebrew translation ended. However, neither the Vatican nor the Christ Church manuscript actually ends at this point.

---

Note 1. Sections of Part Three of al-Hassar's Kitāb al-Bayān, as outlined above, and their manuscript sources.

3.1	Christ Church, fols. 18v to 19r; Vatican, fols. 42r to 44r; Suter’s Drittes Kapitel, pp. 28-29.
3.2	Suter’s Viertes Kapitel, pp. 29-34. There is, however, no break or new heading at this point in the Hebrew texts.
3.2.1	Christ Church, fols. 19r to 20r; Vatican, fols. 44r to 46r; Suter, p. 29.
3.2.2	Christ Church, fols. 20r to 21r; Vatican fols. 46r to 48v; Suter, pp. 29-31.
3.2.3	Christ Church fols. 21r to 22v; Vatican fols. 48v to 52r; Suter pp. 31-34.
3.2.4	Christ Church fol. 22v; Vatican fol. 52r.
3.3	Suter’s Fünftes Kapitel pp. 34-35.
3.3.1	Christ Church fols. 22v to 23r; Vatican fols. 52r to 54r. There are 12 sub-sections.
3.3.2	Christ Church fols. 23r to 24r; Vatican fols. 52r to 54r.
3.3.3	Christ Church fol. 24r; Vatican fol. 55v.
3.4	Suter’s Sechtes Kapitel pp. 35-37.
3.4.1	Christ Church fols. 24r to 26v; Vatican fols. 56r to 61v. The headings of the 22 sub-sections are listed at the end of the section on fols. 26v and 61v, respectively. The text of sub-sections 16 to 21 is missing, however, in the Christ Church manuscript; an entry in the left-hand margin of fol. 26r opposite where they should appear reads בכאן חסר (there are [items] missing here).
3.4.2	Christ Church fols. 26v to 29r; Vatican fols. 61v to 67v.
3.4.3	Christ Church fol. 29v; Vatican fols. 68v to 69v; Suter p. 36.
3.4.4	Christ Church fol. 29v; Vatican fol. 69v; Suter pp. 36-37.
3.5	Suter’s Siebentes Kapitel pp. 37-39.
3.5.1	Christ Church fols. 30r to 31r; Vatican fols. 69v to 72r; Suter p. 37.
3.5.2	Christ Church fols. 31r to 31v; Vatican fols. 72r to 73r; Suter pp. 37-39.

Note 2. Depending on the context, the Hebrew word חיתום can mean Algebra or, as in the present instance, refer to a procedure for augmenting a fraction. In all the instances, the corresponding Arabic word is el-ğebr.

Note 3. For another example see: Friedrich Katscher's article, "Extracting Square Roots Made Easy: A Little Known Medieval Method," and especially the section, “Al-Hassar’s Description of the Method,” here in MAA Convergence. The example Katscher describes is on fol. 31r of the Christ Church manuscript and fol. 73r of the Vatican text.

In the Vatican manuscript of al-Hassar's Kitab al Bayan, this worked example of approximating a square root is followed by a further nine examples and exercises, only after which the colophon appears on fol. 76r (see Note 1). In the Christ Church manuscript, the order is reversed and the same nine examples and exercises appear immediately after the colophons, starting at the top of the left-hand column of fol. 31v and continuing up to fol. 33r (see Figure 2). They do not, however, appear in Suter’s 1901 translation nor, by implication, in the Gotha manuscript.

The presence of these items at the end of the Vatican and Christ Church manuscripts is anomalous. Judging by their subject matter, they really belong much earlier in the text. The first seven are arithmetical calculations involving fractions and belong in the relevant sections of Parts Two and Three. The subject of the eighth item is a long multiplication and that of the ninth is the technique of “casting out nines” used to check the result of a multiplication, both of which really belong under the heading “Multiplication” in Part One. A method for carrying out long multiplications does in fact appear under that heading but it is very different from the one presented here at the end of the Vatican and Christ Church manuscripts.

The method of long multiplication from Part One is the one that was employed when working with a sandboard and involves the deletion and rewriting of numerals at each step; this is easily done on such a device though it can be somewhat confusing and prone to errors. The worked example given is the multiplication of \(43\) by \(76\) and reads as follows (Note 2).

When it is said to you, multiply \(43\) by \(76.\) Put the \(43\) in one row and write the \(76\) in the row below, in such a way that the units column of the second number is under the tens column of the first, in the following manner: \[\genfrac{}{}{0pt}{}{\phantom{7}43}{76\phantom{3}}\]

Now multiply the last digit of the upper number with the first of the lower, i.e., \(4\) with \(7\); this gives \(28.\) Place the \(8\) above the \(7\) in the top line and the \(2\) to its left: \[\genfrac{}{}{0pt}{}{2843}{\phantom{2}76\phantom{3}}\]

Now multiply the same \(4\) by the \(6\) below it, which gives \(24\); superimpose the \(4\) on the upper line (which leaves it unchanged) and add the \(2\) to the \(8\) above the \(7,\) which gives \(10\); delete the \(8\) and put in its place the zero from the ten; add the \(2\) to this and the one from the ten which gives \(3\); delete the \(2\) and put the \(3\) in its place: \[\genfrac{}{}{0pt}{}{2843}{\phantom{2}76\phantom{3}}\rightarrow\genfrac{}{}{0pt}{}{2043}{\phantom{2}76\phantom{3}}\rightarrow\genfrac{}{}{0pt}{}{3043}{\phantom{2}76\phantom{3}}\]

Move the lower number one place to the right so that the \(6\) is under the \(3\) and the \(7\) under the \(4\); then multiply the \(3\) from the upper number by the \(7\) below which gives \(21\); add to this the \(4\) from the upper row which gives \(25\); now delete the \(4\) and set in its place the \(5\); put the \(2\) in the place of zero: \[\genfrac{}{}{0pt}{}{3043}{\phantom{30}76}\rightarrow\genfrac{}{}{0pt}{}{3253}{\phantom{32}76}\]

Multiply the \(3\) by the \(6\) below it which gives \(18\); replace the \(3\) with the \(8\) and add the \(1\) to the \(5\) which gives \(6\); delete the \(5\) and put the \(6\) in its place: \[\genfrac{}{}{0pt}{}{3253}{\phantom{32}76}\rightarrow\genfrac{}{}{0pt}{}{3268}{\phantom{32}76}\] So the result of the multiplication is \(3268.\)

This method became obsolete with the introduction of paper from the Islamic world into medieval Europe and, by the fifteenth century, when the Vatican and Christ Church manuscripts were written, there was clearly a need for a better technique (algorithm), especially for multiplying large numbers. (In fact, Suter (1901, p. 17) expresses surprise that there is no reference to the Lattice Multiplication or to any other method in the Gotha manuscript, attributing this to al-Hassar’s continued use of a sandboard or to copyists’ omissions.) Accordingly, the eighth item in the addendum to the Vatican and Christ Church manuscripts begins: “On the multiplication of integers by another method that is not from the book”: the “book” is presumably al-Hassar’s treatise. What follows is the now familiar pen and paper method of long multiplication. Two worked examples are given: squaring twenty-two, \(22\times 22 = 484,\) and multiplying four hundred and thirty-two by three hundred and twenty-three, \(432\times 323 = 139536.\)

This is as far as the Vatican manuscript goes, but the copyist of the Christ Church manuscript added an example of the lattice (gelosia or sieve) technique for the multiplication of large numbers; in this instance, to calculate the square of the number \(56742\) (see Figure 9).

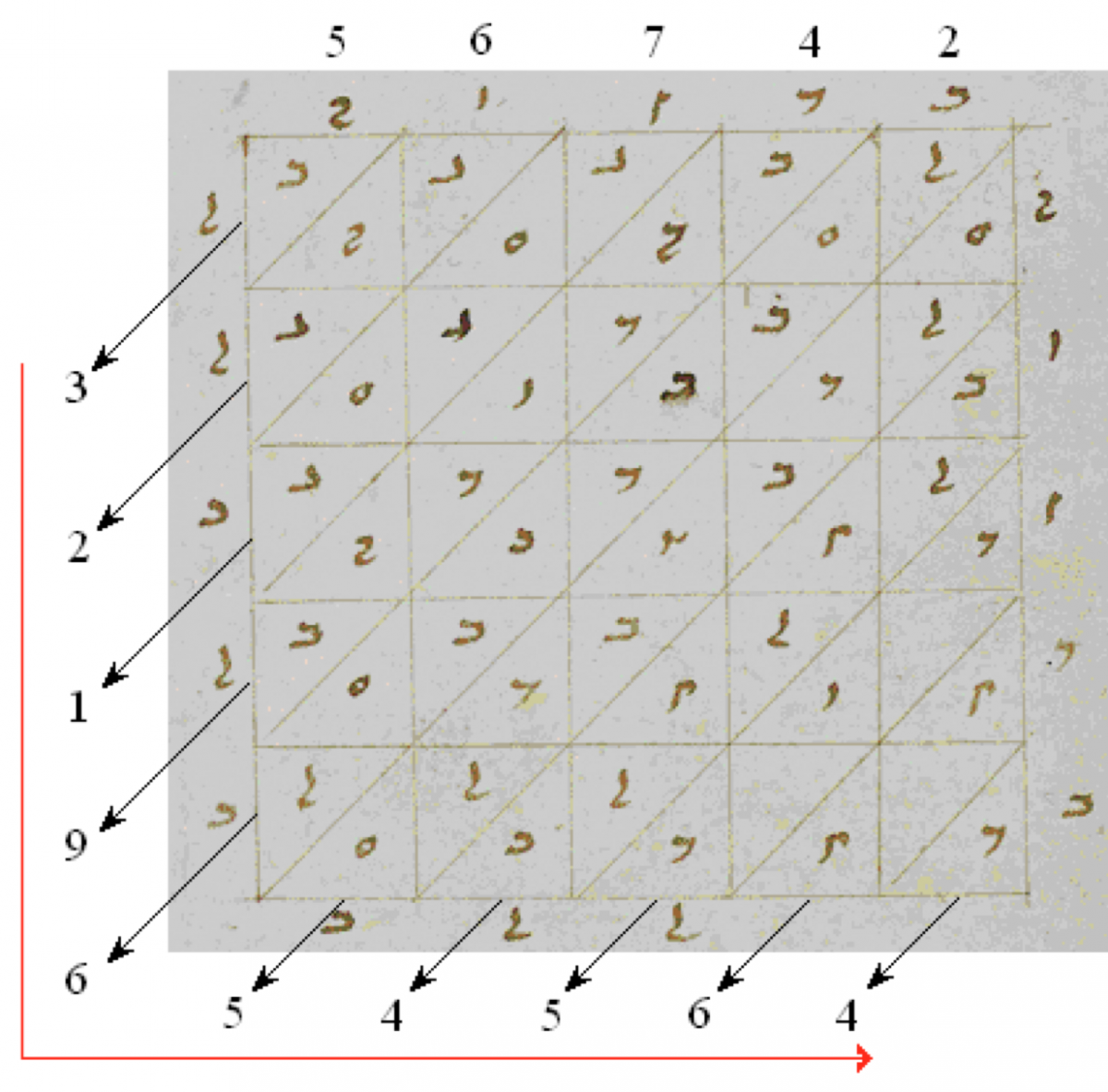
Figure 9. The 5 x 5 grid on fol. 33r of the Christ Church manuscript for calculating the square of \(56742,\) annotated to show how the multiplication is carried out. The multiplicand is across the top of the lattice and the multiplier down the right side; in this example both are the same. A product is calculated for each cell by multiplying the digit at the top of the column and the digit at the right of the row: the tens digit of the product is placed above the diagonal that passes through the cell, and the units digit below. After filling all the cells, the digits in each diagonal are summed, starting from the bottom right cell and the units digit of the sum is entered below the adjacent column, as shown; if the sum is greater them ten, the tens are carried into the next diagonal (written outside the grid at the bottom of each diagonal). After summing all the diagonals, the answer, \(3219654564,\) is read off from top to bottom on the left and continuing from left to right below the grid. (The copyist explained and carried out the procedure correctly but, for some unexplained reason, he entered an incorrect answer, \(22106564,\) in the text.) (Image used by permission of Christ Church College Library)

Fibonacci is often credited with introducing this technique into Europe but this is incorrect. What he described in Chapter 3 of his Liber Abaci is a related technique known as “chessboard multiplication” that works differently. The cells are not divided diagonally and only the lower-order digit is entered in each cell. The earliest extant example of the lattice technique in Europe is in a 14th century Latin manuscript, Tractatus de minutis philosophicis et vulgaribus (A Treatise on Small Measurements, Scientific and General; see Note 3). It also appears in the earliest printed mathematics book, the Treviso Arithmetic, published in the town of that name in 1478, two years after the date of the Christ Church manuscript.

By the fifteenth century, the abacus and Roman numerals that had been in common use for more than a thousand years, were being replaced across Europe by the algorithm and Gobar-based numerals. The transition was slow in coming; the “abacists” would not surrender to the “algorists” without a fight (see Figure 10).

Figure 10. Abacist vs. Algorismist by Gregor Reisch, Margarita philosophica, Strasbourg, 1504. The woodcut shows Arithmetica observing an algorist and an abacist. She appears to favour the algorist; her dress is adorned with Gobar-based numerals and she is looking approvingly in his direction. (See Note 4. This image is provided courtesy of the Columbia University Libraries and may be used for instructional purposes; for all other uses, please contact the Columbia University Libraries. For five more images from the Margarita philosophica (or Pearl of Wisdom), see the article, “Mathematical Treasures – Margarita philosophica of Gregor Reisch,” here in MAA Convergence.)

The advantages of calculating with pen and paper were not always immediately apparent. The abacists’ archaic modes of doing arithmetic would, however, ultimately prove inadequate in the expanding mercantile economies of the Renaissance and this, together with the falling price of paper and the concomitant spread of printing, would ultimately lead to the triumph of the algorists in the sixteenth century.

---

Note 1. In the Vatican manuscript, there is also an appendix on the subject of the extraction of cube roots that is found in neither the Christ Church codex 189 nor Suter’s translation.

Note 2. This example occurs at Christ Church manuscript, fol. 3v and Vatican manuscript, fol. 7v. For another example of this method, see: Episodes in the Mathematics of Medieval Islam, by J.L. Berggren, Springer, New York (1986), p. 34.

Note 3. Bodleian Library, Oxford, MS Digby 190, fol. 75r.

Note 4. For an amusing demonstration of their relative advantages, see Richard Feynman’s “The Abacist versus the Algorist”: http://press.princeton.edu/chapters/i9662.pdf.

Manuscripts

Al-Hassar’s Kitab al-Bayan wa-l-tadhkar

Hebrew

Vatican Library, Rome: Vat. Ebr. 396. Paper, in quarto (ff. 78); Institute of Microfilmed Hebrew Manuscripts (IMHM), National Library of Israel, Jerusalem: Film No. F 474.

Christ Church College Library, Oxford University: Ms. 189. Paper, in folio (ff. 34); Neubauer OX 2457; IMHM, Film No. F 15581. Available as of August 2017 in digital format: http://digital.bodleian.ox.ac.uk/inquire/p/84d98448-57b9-4e7e-a627-9cd2dcc6dd3c

Russian State Library, Moscow: Guenzburg 30; IMHM Film No. F 6711; fols. 124r to 189r.

Arabic

Lawrence J. Schoenberg Collection, Rare Book & Manuscript Library, University of Pennsylvania, LJS 293: http://dla.library.upenn.edu/dla/medren/detail.html?id=MEDREN_4819340

“Abu Muhammed bin Abdullah bin Ayyash known as Hassar … Kitab al-Bayan Wa al-Tidhkar.” Christies of South Kensington, Lot 146; Sale Date April 18, 2016: http://www.christies.com

Another manuscript

Tractatus de minutis philosophicis et vulgaribus (A Treatise on Small Measurements, Scientific and General), Bodleian Library, Oxford, MS Digby 190.

Books and Articles

Aballagheta, M. and A. Djebbar, Découverte d’un écrit mathématique d’al-Ḥ̣̣aṣṣâr (Xlle S.): Le livre I du Kamil, Historia Mathematica 14 (1987), 147-158.

Berggren, J. L., Episodes in the Mathematics of Medieval Islam, Springer, New York (1986).

Briquet, Charles M., Les Filigranes. Paris etc. 1907.

Djebbar, Ahmed, Mathematics in Medieval Maghreb, Amuchma Newsletter-15 [African Mathematical Union], Universidade Pedagógica (UP), Maputo, Mozambique (1995): http://www.math.buffalo.edu/mad/AMU/amu_chma_15.html

Feynman, Richard, “The Abacist versus the Algorist”: http://press.princeton.edu/chapters/i9662.pdf

Karpinski, L. C., The History of Arithmetic, Rand McNally, Chicago, 1925.

Katscher, Friedrich, “Extracting Square Roots Made Easy: A Little Known Medieval Method.” MAA Convergence, vol. 7 (Nov. 2010), DOI:10.4169/loci003494.

Kunitzsch, Paul, “A New Manuscript of Abu Bakr al-Hassar's Kitab al-Bayan.” Suhayl, International Journal for the History of the Exact and Natural Sciences in Islamic Civilisation 3, 2002-03, S.187-192: http://www.ub.edu/arab/suhayl/volums/volum3/paper%203.pdf

Kunitzsch, Paul, “Al-Hassâr's Kitâb al-Bayân and the Transmission of the Hindu-Arabic Numerals.” Muslim Heritage: http://www.muslimheritage.com/topics/default.cfm?ArticleID=705

Lamrabet, Driss, Introduction à l’histoire des mathématiques maghrébines, Rabat, 1994, 2013.

Menninger, Karl, Number Words and Number Symbols, Dover Publications, 1992.

Miller, Jeff, “Earliest Uses of Symbols for Fractions,” last updated Dec. 22, 2014, in Earliest Uses of Various Mathematical Symbols: http://jeff560.tripod.com/mathsym.html

Pertsch, Wilhelm: Theil 3, Bd. 3: Die Arabischen Handschriften der Herzoglichen Bibliot [...], Gotha, 1881: http://archive.thulb.uni-jena.de/ufb/receive/ufb_cbu_00002974

Saidan, A. S., The Arithmetic of Al-Uqlidisi, D. Reidel Publishing Co., Dordrecht-Holland (1978). Includes English translation of a 12th century copy of the Kitāb al-Fusūl fī al-Ḥisāb al Hindī, written by Abū al Ḥasan al Uqlīdisī in Damascus in 952 CE.

Selin, Helaine, Encyclopaedia of the History of Science, Technology and Medicine in Non-Western Cultures, Springer Science+Business Media, Dordrecht (1997).

Steinschneider, Moritz, Die Hebraeischen Ubersetzungen des Mittlealters…, Berlin 1893.

Suter, H., “Das Rechenbuch des Abu Zakarîjâ el-Ḥ̣̣aṣṣâr,” Bibliotheca Mathematica, 3. Folge 2. Band 1901, pp. 12-40.

Suter, H., “Abhandlungen zur Geschichte der mathematischen Wissenschaften mit Einschluss ihrer Anwendungen” (Discourses on the history of mathematical sciences, including their applications), Druck und Verlag von B. G. Teubner, Leipzig (1900): http://quod.lib.umich.edu/u/umhistmath/ACD4271.0001.001?rgn=main;view=fulltext

Swetz, Frank J. and Victor J. Katz, “Mathematical Treasures – Margarita philosophica of Gregor Reisch,” MAA Convergence.

Zonghi’s Watermarks, The Paper Publication Society, Hilversum, 1953.

Further reading

Berggren, J. Lennart, “Chapter 3. Mathematics in the Islamic World in Medieval Spain and North Africa,” in Victor J. Katz (ed.), Sourcebook in the Mathematics of Medieval Europe and North Africa, Princeton University Press, 2016, pp. 381-547.