More Classroom Activities Based on Ancient Indian Rope Geometry
Introduction
One source of mathematics from ancient India is a group of texts known as the Śulba-sūtras, which were written between 800 and 350 BCE (a timeline can be found at the MacTutor History of Mathematics Archive). These works contained instructions for building a variety of altars for fire sacrifices. The name of the texts, Śulba-sūtra, translates as “rules of the cord.” The four best known Śulba-sūtras, named after their authors and listed in order of importance, are Baudhāyana-śulba-sūtra, Āpastamba-śulba-sūtra, Kātyāyana-śulba-sūtra, and Mānava-śulba-sūtra (for more information on the history of the Śulba-sūtras and Vedic rituals, see [Plofker1], [Plofker2], and [Prasoon]). These fire altar manuals, with instructions for laying out altars of fixed area in particular shapes and then filling them in with a fixed number of bricks of various shapes, necessarily contained much mathematics. Mathematical ideas included measurement, constructing right angles, transforming from one geometric shape to another such as square to rectangle or trapezoid, approximating irrational numbers like \(\sqrt 2\) and \(\pi,\) working with fractions, and an equivalent of the Pythagorean theorem prior to Pythagoras.
Several GeoGebra applets and other classroom activities based on the Śulba-sūtras can be found in a previous article by the authors, "Ancient Indian Rope Geometry in the Classroom" [Huffman and Thuong], here in Convergence. The current article is a continuation with additional GeoGebra applets and activities based on the translation found in [Sen and Bag] of the Baudhāyana-śulba-sūtra, which we will abbreviate BSS. We follow the labelling and notation of the Sen and Bag translation. For example, BSS 1.6 will refer to Stanza 1, Verse 6. Words in parentheses in the translations throughout the article and applets have been added by the translator to aid the reader. It should be noted that the original text is very terse with no diagrams or verification of the correctness of the mathematics.
The Baudhāyana-śulba-sūtra is the most complete of the Śulba-sūtras, and is also considered by many to be the most important. It begins with a brief description of some units of measurement before providing two different methods for constructing a square. The square is the basis for the fire altar constructions. The first square construction method in the BSS incorporates constructing a perpendicular bisector, while the second method uses the converse of the Pythagorean Theorem to form right angles (see Figure 1). In their previous article, the authors have provided an activity for elementary and middle school students that involves measurement using three units of length from ancient India, as well as both indoor and outdoor student activities (see "Ancient Indian Rope Geometry in the Classroom – Student Activities") based on Baudhāyana’s first method of constructing a square. Indoor and outdoor student activities using the second method in BSS are included at the end of this article.
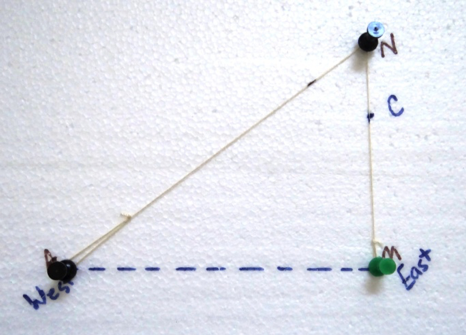
Figure 1. A 3-4-5 triangle is used to form a right angle in the authors' second method of constructing a square.
After the methods for constructing squares, the Baudhāyana-śulba-sūtra (BSS) provides methods for constructing rectangles, and for transforming squares into various shapes. GeoGebra applets for adding and subtracting squares, transforming a rectangle into a square of equal area, and approximating the square root of two are provided in the authors' "Ancient Indian Rope Geometry in the Classroom" [Huffman and Thuong] here in Convergence. The present article contains GeoGebra applets for two constructions and four more transformations from BSS. The two constructions are for a rectangle (BSS 1.6), and for a square (BSS 1.8). The four transformations are square to rectangle (BSS 2.3–2.4), rectangle to trapezoid (BSS 2.6), rectangle to triangle (BSS 2.7), and square to circle (BSS 2.9). Proofs are provided for each applet, but students could be shown the applets only and asked to prove themselves that the construction or transformation actually yields the desired shape.
More Classroom Activities Based on Ancient Indian Rope Geometry - Constructing a rectangle
The following applet demonstrates the method for constructing a rectangle of given dimensions found in Section 1.6 of the Śulba-sūtra of Baudhāyana (BSS 1.6). In the applet, slide \(E\) to change the side length of the desired rectangle and \(A\) to change the desired width (in step 3). The translated text is in black, with corresponding interpretation in blue below it. Click on “Go” to advance through the construction, and “Reset” when the construction is completed.
In BSS 1.6, Baudhāyana presented a method to construct a rectangle using only ropes and stakes in the ground [Sen and Bag, p. 78]:
When (the construction of) a rectangle is desired, two poles are fixed on the ground at a distance equal to the desired length. (This makes the east-west line.) Two poles one on each side of the (two above mentioned) poles are fixed at equal distances (along the east-west line). A cord equal in length to the breadth (of the rectangle) is taken, its two ends are tied and a mark is given at the middle. With the two ties fastened to the end poles (on either side of the pole) in the east, the cord is stretched to the south by the mark; at the mark (where it touches the ground) a sign is given. Both the ties are now fastened to the middle (pole at the east end of the prāci), the cord is stretched towards the south by the mark over the sign (previously obtained) and a pole is fixed at the mark. This is the south-east corner. In this way are explained the north-east and the two western corners (of the rectangle).
Here is an explanation of the method quoted above. First, we begin with two stakes in the ground at points \(E\) and \(W.\) The length of \(EW\) shall be the length of the desired rectangle and can be adjusted in the applet by sliding point \(E.\)
In step 2 of the applet, two points \(Q_1\) and \(P_1\) are fixed at equal distance from \(E\) to the left and right, respectively, and collinear to the east-west line. The distance is arbitrary and can be adjusted by sliding point \(P_1\) in the applet. This is easy to do with ropes in the ground since it just involves extending segment \(EW\) and measuring appropriate distances.
In step 3, a rope of length equal to the desired width is cut, and its midpoint is marked. This is represented by segment \(AB\) with midpoint \(N.\) To adjust the desired width, slide point \(A\) in the applet. In step 4, we attach the ends of the rope \(A\) and \(B\) to points \(Q_1\) and \(P_1,\) respectively, and pull \(N\) south until the rope is taut. We mark point \(S_1\) where \(N\) ends up. It is important to note that \(S_1\) is not a corner of the rectangle. It is merely an auxiliary point that ensures angle \(WES_1\) is a right angle. To see that \(WES_1\) is a right angle, note that triangles \(EQ_1S_1\) and \(EP_1S_1\) are congruent by construction, so that angles \(Q_1ES_1\) and \(P_1ES_1\) are equal. They must sum to a straight angle of 180 degrees and hence they are both 90 degrees.
Now corner \(G\) is marked by attaching both ends of rope \(AB\) to point \(E\) and pulling on \(N\) until the rope is taut. Where point \(N\) ends up is the location of \(G.\) The remaining three corners are found in a similar fashion, resulting in rectangle \(CDGF.\) By construction, \(EG\) and \(DE\) are half of the desired side width, and hence \(DG\) has length equal to the desired width. Similarly, \(CF\) also has the desired width as length. Because angles \(WED,\) \(WEG,\) \(EWC,\) and \(EWF\) are all 90 degrees, so are all the required angles at \(C,\) \(D,\) \(F,\) and \(G.\) This can be seen by observing \(CD\) and \(FG\) are parallel to \(EW\) by construction.
It is interesting to note that this method does not rely on the Pythagorean Theorem, and can be used to construct a square when \(AB\) has the same length as \(EW.\)
More Classroom Activities Based on Ancient Indian Rope Geometry - Constructing a square
The following applet demonstrates the method for constructing a square found in Section 1.8 of the Śulba-sūtra of Baudhāyana (BSS 1.8). Slide \(E\) to change the side length of the desired square. The translated text is in black, with corresponding interpretation below it in blue. Click on “Go” to advance through the construction, and “Reset” when the construction is completed.
Baudhāyana provided a method for constructing a square in BSS 1.8 [Sen and Bag, p. 78]:
Now another (method). Ties are made at both ends of a cord of length equal to the measure increased by its half (so that the whole length of the cord is divided into three parts of half the measure each). In the third [extended] part on the western side a mark is given at a point shorter by one-sixth (of the third part); this is the nyañcana. Another mark is made at the desired point for fixing the corners. With the two ties fastened to the two ends of the east-west line (prsthyā), the cord is stretched towards the south by the nyañcana, and the western and eastern corners [of the square] are fixed by the desired mark.
Baudhāyana’s construction of a square in BSS 1.8 using only ropes and stakes in the ground makes implicit use of the converse of the Pythagorean Theorem (pre-Pythagoras) to ensure that all angles are indeed right. To begin the construction, a rope of length equal to the desired side length of the square is laid along the east-west line. The desired length can be adjusted by moving \(E\) in the applet. Thus, our goal is to construct a square with side length \(EW.\)
In step 1 of the applet, the rope is extended to three-halves of the desired length. That is,
\[WZ={\frac{3}{2}}EW,\]
while
\[EZ={\frac{1}{2}}EW.\]
Next a mark is made on the rope “at a point shorter by one-sixth (of the third part); this is the nyañcana...” So in step 2 of the applet we have defined point \(N\) on the rope so that \(EN\) is one-twelfth of the original side length. Notice that the “third” part is segment \(EZ\) which is half of the desired side length, and one-sixth of one-half is indeed one-twelfth: \[EN={\frac{1}{6}}EZ={\frac{1}{6}}\cdot{\frac{1}{2}}EW={\frac{1}{12}}EW.\]
Moreover,
\[NZ=EZ-EN={\frac{1}{2}}EW-{\frac{1}{12}}EW={\frac{5}{12}}EW.\]
In step 3, the midpoint of segment \(EW\) is marked. This will be important in the construction later.
In step 4, we see use of the converse of the Pythagorean Theorem. The applet simulates fixing stakes in the ground at point \(W\) and \(E.\) Imagine taking the rope of length \(WZ\) and attaching its ends to \(W\) and \(E,\) respectively. Pull point \(N\) to the south until the rope is taut. It should be noted that when point \(N\) is pulled directly south, there will be a unique point at which the rope is taut. We denote this point \(Q_1\) in the applet. So, in the applet, when \(N\) is pulled south until it is taut, point \(Z\) coincides with \(E,\) and \(N\) coincides with \(Q_1.\) Thus, \(EQ_1=ZN\) and \(Q_1W=NW.\) Now we claim that triangle \(EQ_1W\) is indeed a right triangle. To see this, note that \[EQ_1=ZN=\frac{5}{12}EW,\] \[Q_1W=NW=EW+EN=EW+\frac{1}{12}EW=\frac{13}{12}EW,\] and therefore:
\[EW^2+EQ_1^2=EW^2+\frac{25}{144}EW^2=\frac{169}{144}EW^2=\left(\frac{13}{12}EW\right)^2=Q_1W^2,\]
or \[EW^2+EQ_1^2 =Q_1W^2.\]
Applying the converse of the Pythagorean Theorem, we infer that angle \(WEQ_1\) is indeed right.
The remaining points \(P_1, Q_2,\) and \(P_2\) are constructed in analogous fashion as shown in the applet, with angles \(WEP_1, EWQ_2,\) and \(EWP_2\) all being right. However, it is important to note that the four points \(Q_1, P_1, Q_2,\) and \(P_2\) are not the four corners of the square, as \(EQ_1, EP_1, WQ_2,\) and \(WP_2\) are \(5/12\)ths of the desired square side length (just a little less than half the desired side length).
In step 5 of the applet, we finally fix the four corners of the square. This is where point \(M\) is used. Take a rope of length \(EW\) (the desired square side length). Recall \(M\) was the midpoint of this rope. Attach both ends of the rope to point \(E\) and pull \(M\) south so that the rope passes through point \(Q_1.\) Now the point where \(M\) ends up determines corner \(A\) of the square. Indeed, this is a simple step, as we are just extending segment \(EQ_1\) to a segment with half the desired side length. The remaining corners \(B,\) \(C,\) and \(D\) are fixed in analogous fashion to obtain square \(ABDC,\) as desired. The fact that angles \(WEQ_1, WEP_1, EWQ_2,\) and \(EWP_2\) are right allow us to infer the angles at \(A,\) \(B,\) \(C,\) and \(D\) are right because sides \(BD\) and \(AC\) are parallel to the east-west line by construction.
More Classroom Activities Based on Ancient Indian Rope Geometry - Transforming a square into a rectangle of equal area
The applet directly below demonstrates the method found in Section 2.4 of the Śulba-sūtra of Baudhāyana (BSS 2.4) for transforming a square into a rectangle of equal area. Click “Go” to advance to the next step and “Reset” when the construction is completed. The dimensions of the given square can be adjusted by sliding points \(A\) and \(D.\) The longer side of the desired rectangle can be adjusted by sliding point \(E.\) Slide point \(P\) to \(E\) to make the area of rectangle \(DFHI\) equal to that of \(ABDC.\)
The problem of transforming a square into a rectangle of equal area was considered by Baudhāyana in Sections 2.3-2.4 of BSS [Sen and Bag, p. 79].
2.3 – A square intended to be transformed into a rectangle is cut off by its diagonal. One portion is divided into two (equal) parts which are placed on the two sides (of the other portion) so as to fit (them exactly).
2.4 – Or else, if a square is to be transformed (into a rectangle), (a segment) of it is to be cut off by the side (of the rectangle); what is left out (of the square) is added to the other side…
It is clear that the intention of BSS 2.3 is to produce a rectangle of equal area, with one side of the rectangle having length equal to the diagonal of the square. The figures below demonstrate this. Say we wish to construct a rectangle with area equal to that of square \(ABDC\) and with one side length equal to the length of the square’s diagonal. The construction is rather simple: just divide the square in two pieces along the diagonal. Mark \(M,\) the midpoint of the diagonal, further dividing triangle \(ABC\) into two congruent triangles \(ABM\) and \(ACM,\) the areas of which are of course one fourth of the area of the square. Then construct congruent triangles \(ABF\) and \(ACE\) (say by reflecting \(ABM\) and \(ACM,\) respectively). The area of rectangle \(BCEF\) evidently equals that of \(ABDC,\) as it is just 4 times the area of \(ABM.\)

Figure 2. Construction of rectangle \(BCEF\) with area equal to that of square \(ABDC\) and one side being the diagonal of the square.
There are challenges in interpreting exactly what Baudhāyana intended in BSS 2.4. The same rule is given in 3.1 of the Śulba-sūtra of Āpastamba, and also with no clear interpretation and missing details (see, for example, the discussion on p. 159 of [Sen and Bag]). The method presented here for transforming a square into a rectangle of any side lengths is due to Datta, and captures the spirit of Baudhāyana’s method [Datta]. This is a more general approach than that found in 2.3 because we have the freedom to choose one of the side lengths of the rectangle.
To begin the construction, start with a square \(ABDC.\) Now there are an infinite number of non-square rectangles with area equal to that of the square, but once we have fixed one of the side lengths, say \(L_1,\) the other side length is of course determined: \[L_2=\frac{{\rm{Area}}(ABDC)}{L_1}.\] Evidently, one side length will be less than the square side length, and one will be greater. Without loss of generality, suppose \(L_1\) is the greater one. Datta’s method allows us to choose the greater side length. First, we extend segment \(AC\) to \(CE,\) so the length of \(CE\) is \(L_1.\) Similarly, extend \(BD\) to \(DF\) so that \(DF\) has length \(L_1.\) We have constructed a rectangle \(CEFD\) which has one side length equal to that of the given square, and one side length equal to the desired side length \(L_1.\) Let \(P\) be a point on side \(EF.\) Extend a rope from point \(P\) to \(D.\) The rope will intersect side \(AB\) at a point, say \(G.\) Now the length of segment \(BG\) is taken to be the second side length of the desired rectangle.
Now consider the red rectangle \(DIHF.\) In the applet, one may slide point \(P\) along segment \(EF\) to see how the area of \(DIHF\) (and position of \(G\)) changes. When point \(P\) meets point \(E,\) we see that \(DIHF\) is the desired rectangle. To prove this, first slide point \(P\) to point \(D,\) and note the area of \(CEFD\) is split into two by segment \(DP:\)
\[\frac{1}{2}{\rm{Area}}(CEFD)= {\rm{Area(Triangle}}(GHP))+{\rm{Area(Rect}}(BGHF))+{\rm{Area(Triangle}}(BDG)).\]
\[\frac{1}{2}{\rm{Area}}(CEFD)= {\rm{Area(Triangle}}(AGP))+{\rm{Area(Rect}}(AGIC))+{\rm{Area(Triangle}}(DIG)).\]
By symmetry,
\[{\rm{Area(Triangle}}(GHP))={\rm{Area(Triangle}}(AGP))\]
and
\[{\rm{Area(Triangle}}(BDG))={\rm{Area(Triangle}}(DIG)).\]
Therefore, combining the above equations allows us to infer
\[{\rm{Area(Rect}}(BGHF))={\rm{Area(Rect}}(AGIC)).\]
Thus \[{\rm{Area}}(DIHF)={\rm{Area}}(BGHF)+{\rm{Area}}(BDIG)={\rm{Area}}(AGIC)+{\rm{Area}}(BDIG)={\rm{Area}}(ABDC),\] as required.
More Classroom Activities Based on Ancient Indian Rope Geometry - Transforming a rectangle into an isosceles trapezoid of equal area
The applet below demonstrates the method found in Section 2.6 of the Śulba-sūtra of Baudhāyana (BSS 2.6) for transforming a rectangle into an isosceles trapezoid of the same area. Click “Go” to advance to the next step and “Reset” when the construction is completed. The dimensions of the given rectangle can be adjusted by sliding points \(A\) and \(D.\) The shorter side of the trapezoid can be adjusted by sliding point \(E.\)
Baudhāyana gave a method for transforming a rectangle into an isosceles trapezoid of area equal to that of the given rectangle. Below is a translation of the original source, which may be found in section 2.6 of BSS [Sen and Bag, p. 79].
If it is desired to reduce one side of a square (that is, to make an isosceles trapezium), the reduced side is to be taken as the breadth (of a rectangular portion to be cut off from the square); the remaining part (of the square) is divided by the diagonal and (one half), after being inverted, is placed on the other side.
First suppose that rectangle \(ABCD\) has been constructed, perhaps by the previously described method. The area of the given rectangle can be adjusted by sliding points \(A\) and \(D.\) First point \(E\) is marked on side \(AB.\) The length of \(AE\) is to be the smaller base of the isosceles trapezoid. Now point \(F\) can be marked on side \(CD,\) so that \(CF\) has the same length as \(AE.\) Note this forces segment \(AC\) to be parallel to segment \(EF\) and hence triangle \(DEF\) is indeed a right triangle.
As suggested by the original source, the next step is to reflect triangle \(DEF\) about a vertical line, and translate left, so that \(EF\) coincides with \(AC,\) thus constructing triangle \(ACG,\) congruent to \(DEF.\) To do this entirely with ropes, take two ropes of lengths \(DE\) and \(DF.\) Attach one end of the rope of length \(DE\) to point \(A,\) and one end of the rope of length \(DF\) to point \(C.\) Now pulling on the unattached ends of both cords until taut (to the left), they will meet at precisely one point: \(G.\)
The desired trapezoid is now marked by \(AEDG.\) To see it has the same area as rectangle \(ABDC,\) note that by congruence of triangles \(ACG,\) \(DEF,\) and \(BDE,\)
\[{\rm{Area}}(AEDG)={\rm{Area}}(ACG)+{\rm{Area}}(AEFC)+{\rm{Area}}(DEF)\]
\[={\rm{Area}}(AEFC)+{\rm{Area}}(DEF)+{\rm{Area}}(BDE)={\rm{Area}}(ABDC).\]
This can also be seen algebraically. The area of a trapezoid is the average of the base lengths multiplied by the height. That is,
\[{\rm{Area}}(AEDG)=AC\cdot\frac{1}{2}(AE+DG).\]
Now observe that \(DG=CG+CF+FD=CF+2\cdot FD,\) and using that \(AE=CF,\)
\[AE+DG=CF+(CF+2\cdot FD)=2(CF+FD)=2\cdot CD.\] Thus,
\[{\rm{Area}}(AEDG)=AC\cdot\frac{1}{2}(AE+DG)=AC\cdot CD.\]
It is important to note too from this formula that once we have fixed the dimensions of the rectangle, \({\rm{Area}}(AEDG)\) and \(AC\) are both fixed. Once \(AE\) is specified, the trapezoid dimensions are determined completely as \(DG\) can be solved for: \(DG=2\cdot CD-AE.\)
It is also interesting to note the degenerate case, when point \(E\) meets point \(A,\) corresponds to simply cutting up rectangle \(ABDC\) along its diagonal into two triangles and rearranging them.
Observe that this construction can be done entirely with ropes and stakes in the ground: the “heavy lifting” was all contained in the construction of the rectangle. Here we did not need to use the converse of the Pythagorean Theorem to infer that \(DEF\) was a right angle.

Figure 3. Construction of a falcon fire altar at an Athirathram ceremony in 2011. Notice the trapezoidal bricks in the lower left corner. (Photo from Wikimedia Commons attributed to Arayilpdas at Malayalam Wikipedia.)
More Classroom Activities Based on Ancient Indian Rope Geometry - Transforming a rectangle into a triangle of equal area
The following applet demonstrates a method, generalized from one in Section 2.7 of the Śulba-sūtra of Baudhāyana (BSS 2.7), for transforming a rectangle into a triangle of equal area. Click “Go” to advance to the next step and “Reset” when the construction is completed. The dimensions of the given rectangle can be adjusted by sliding points \(A\) and \(D.\) Slide point \(X\) to \(X^{\prime}\) to make the area of triangle \(RTY\) equal to the area of \(ABDC.\) Slide point \(Y\) to \(Y^{\prime}\) to make the triangle isosceles.
Baudhāyana considered the problem of transforming a square into an isosceles triangle of equal area in section 2.7 of BSS. The original translation is below [p. 79, Sen and Bag].
If it is desired to transform a square into (an isosceles) triangle, the square whose area is to be transformed is doubled and a pole fixed at the middle of its east side; two cords with their ties fastened to it (the pole) are stretched to the south-western and north-western corners (of the square); portions lying outside the cords are cut off.
Here we will present a solution to the more general problem of transforming a rectangle into a triangle (not necessarily isosceles) of equal area. Suppose we have constructed rectangle \(ABDC,\) say with the previously described method in this article. Next construct rectangle \(EFHG,\) with twice the height of \(ABDC.\) This is of course easy to do with ropes and stakes once \(A\) is constructed. Note the area of \(EFHG\) is twice that of \(ABDC.\)
Now we find a square with area equal to that of the doubled rectangle. To do this, we appeal to the method found in Section 2.5 of Baudhāyana’s Śulba-sūtra, or equivalently, the method found in Section 2.7 of Āpastamba's Śulba-sūtra. First mark points \(P_4\) and \(P_5\) so that \(HGP_4P_5\) forms a square. Now add half of the length of segment \(EP_4\) onto \(GH\) to make segment \(GT.\) Now extend square \(HGP_4P_5\) to an “auxiliary square” with side \(GT,\) with other points \(P_i\) as indicated. Now consider a semicircular arc with center \(T\) and radius equal to the length of \(GT\) (the auxiliary square side length). Let \(X\) denote an arbitrary point on this arc. As shown, \(X\) determines a square \(QRTS.\) When \(X\) reaches \(X^{\prime},\) the area of \(QRTS\) is equal to the area of \(EFHG\) (which is twice that of rectangle \(ABDC\) that we started with).
The proof that \({\rm{Area}}(QRTS)={\rm{Area}}(EFHG)\) when \(X^{\prime}\) reaches \(X\) relies on the Pythagorean Theorem. Indeed, assume that \(X^{\prime}\) and \(X\) coincide. We can divide rectangle \(EFHG\) into three smaller sub-rectangles, thus obtaining
\[{\rm{Area}}(EFHG)={\rm{Area}}(EFP_2P_1)+{\rm{Area}}(P_1P_2P_5P_4)+ {\rm{Area}}( P_4P_5HG).\]
We can divide square \(P_1P_3TG\) into two sub-rectangles and two sub-squares with
\[{\rm{Area}}( P_1P_3TG)={\rm{Area}}(P_1P_2P_5P_4)+{\rm{Area}}( P_5P_6TH)+ {\rm{Area}}( P_4P_5HG)+{\rm{Area}}(P_2P_3P_6P_5).\]
Further, by symmetry, \({\rm{Area}}(P_1P_2P_5P_4)={\rm{Area}}(EFP_2P_1),\) which allows us to infer \[{\rm{Area}}( P_1P_3TG)={\rm{Area}}(EFHG)+{\rm{Area}}(P_2P_3P_6P_5).\]
Thus, this becomes a problem of “subtracting squares.” We seek a square with area equal to \[{\rm{Area}}( P_1P_3TG)-{\rm{Area}}(P_2P_3P_6P_5).\] Observe that \[{\rm{Area}}(P_2P_3P_6P_5)=HT^2\] and \[{\rm{Area}}( P_1P_3TG)=P_3T^2=XT^2.\]
The last equalities follow since \(P_3T\) and \(XT\) are radii of the same circular arc. By the Pythagorean Theorem applied to right triangle \(XTH,\) \(XH^2+HT^2=XT^2,\) so that
\[XH^2=XT^2-HT^2={\rm{Area}}( P_1P_3TG)-{\rm{Area}}(P_2P_3P_6P_5)= {\rm{Area}}(EFHG).\]
Finally observe that \(XH\) is the side length of \(QRTS.\) (For more details consult "Ancient Indian Rope Geometry in the Classroom" [Huffman and Thuong].) So we have \[{\rm{Area}}(QRTS)= {\rm{Area}}(EFHG)=2\cdot{\rm{Area}}(ABDC).\]
As a matter of fact, this was the most difficult part of the construction. Now take any point \(Y\) on side \(QS.\) The area of triangle \(RTY\) is half the area of \(QRTS.\) To see this, note that its base and height are both equal to the side length of \(QRTS,\) so that \[{\rm{Area}}(RTY) =\frac{1}{2}{\rm{(Base)(Height)}} =\frac{1}{2}{\rm{Area}}(QRTS)={\rm{Area}}(ABDC).\]
To make triangle \(RTY\) isosceles, simply slide point \(Y\) to \(Y^{\prime},\) the midpoint of segment \(QS.\)

Figure 4. A soma cart with an isosceles triangle forepart from an Agnicayana fire sacrifice ritual in 2011 in Panjal, Kerala. (Photo from Wikimedia Commons: https://commons.wikimedia.org/wiki/File:Soma_cart_used_in_Athirathram_2011.JPG)
More Classroom Activities Based on Ancient Indian Rope Geometry - Transforming a square into a circle
This applet demonstrates the procedure found in Section 2.9 of the Śulba-sūtra of Baudhāyana (BSS 2.9) for transforming a square into a circle of equal area. The construction actually produces a circle (shown in blue) with area approximately equal to that of the square. The circle with area exactly equal to that of the square is shown in pink. The actual construction of the circle with area exactly equal to that of the square is impossible using only straightedge and compass, a consequence of the transcendental nature of \(\pi.\) Click “Go” to advance through the construction and “Reset” when the construction is completed.
Finally, we now turn our attention to the method that Baudhāyana gave in BSS 2.9 to construct a circle with area approximately equal to that of a given square. The translated original source is as follows [Sen and Bag, p. 4]:
If it is desired to transform a square into a circle, (a cord of length) half the diagonal (of the square) is stretched from the center to the east (a part of it lying outside the eastern side of the square); with one-third (of the part lying outside) added to the remainder (of the half diagonal), the (required) circle is drawn.
This method only gives an approximate answer. In the applet, consider square \(ABCD,\) the dimensions of which can be adjusted by sliding point \(A.\) First a rope is extended from the center of the square, \(O,\) to the corner \(B.\) The length of this rope is of course half the diagonal of the square. Keeping one end of the rope attached to \(O,\) pulling the other end southward traces out arc \(BEA.\) Let \(E\) be the point of the arc that points toward the east, and let \(G\) be the intersection with the side \(AB\) of the square.
As suggested by the translation, next, point \(P\) is marked so that \(GP\) is one-third of \(EG:\) \[GP=\frac{1}{3}EG.\]
In the next step, we define point \(F\) to be a point on segment \(EP.\) Slide point \(F\) to observe how the area of the blue circle with radius \(OF\) changes. When point \(F\) reaches point \(P,\) the area of the blue circle will have area only approximately equal to that of \(ABCD.\)
Point \(Z\) is marked so that a circle of radius \(OZ\) has area exactly equal to that of \(ABCD.\) The reader may observe that the blue approximate circle is just slightly bigger than the pink exact circle. We now calculate just how good of an approximation Baudhāyana gave.
Let \(L\) denote half the side length of \(ABCD.\) Thus the length of \(OG\) is equal to \(L.\) The length of \(BO\) (and hence that of \(EO\)) is \({\sqrt{2}}L.\) Hence the radius that Baudhāyana gave is \[OP=OG+GP=OG+\frac{1}{3}EG=L+\frac{1}{3}\left({\sqrt{2}}L-L\right)=\frac{2+\sqrt{2}}{3}L.\]
To find the exact radius, note that the area of \(ABCD\) is \((2L)^2=4L^2.\) Solving \({\pi}r^2=4L^2\) yields an exact radius of \[r=\frac{2}{\sqrt{\pi}}L.\]
Comparing the coefficients on \(L,\) we see that \[\frac{2+\sqrt{2}}{3}=1.138071\dots\quad{\rm{while}}\quad\frac{2}{\sqrt{\pi}}=1.128379\dots.\]
This amounts to a relative error of less than 1%. Note that we can also infer an approximation of \(\pi\) from this calculation. We solve for \(\pi\) in \[\frac{2+\sqrt{2}}{3}\approx\frac{2}{\sqrt{\pi}}\] to obtain \[\pi\approx\left(\frac{6}{2+\sqrt{2}}\right)^2.\]
Baudhāyana derived a rational estimation of \(\sqrt{2}\) as \[\frac{577}{408}=1.414215686\dots\] in Section 2.12 of BSS. (See "Ancient Indian Rope Geometry in the Classroom" [Huffman and Thuong] for an exposition.) Thus we obtain the rational approximation of \(\pi:\) \[\pi\approx\left(\frac{6}{2+\frac{577}{408}}\right)^2=\left(\frac{2448}{1393}\right)^2=3.0883\dots.\]
More Classroom Activities Based on Ancient Indian Rope Geometry - Student Activities
Activity 1. Constructing a Square an Ancient Indian Way (Inside Version)
This activity can be used with middle school or high school students. Using cardboard, string, and pushpins, students model one of the several ways for constructing a square used in ancient India in the building of a fire altar. Common Core Standard G.CO.12 (http://www.corestandards.org/Math/Content/HSG/CO/D/12/) recommends making formal geometric constructions with a variety of tools and methods.
Activity 2. Constructing a Square an Ancient Indian Way (Outside Version)
The above activity can be completed outside in a more realistic fashion, using pegs or stakes instead of pushpins and a rope or cord instead of the string. If there is no open ground available, the activity can be completed on a parking lot using sidewalk chalk, with students holding the rope in place instead of pounding pegs into the ground.
Activity 3. Constructing a Square Fire Altar
This activity provides an application of solving a system of two linear equations in two unknowns for students in an algebra course. It follows instructions for constructing a square gārhapatya fire altar from BSS 7.4-7.7. Since the instructions for constructing the specific fire altar are somewhat vague, the activity allows students to explore constructing a square altar with square paper “bricks” and to discover arrangements themselves.
More Classroom Activities Based on Ancient Indian Rope Geometry - Conclusion and Resources
Conclusion
Students can benefit from exploring original sources in mathematics, and the ancient Śulba-sūtras texts from India are a great source of applications of mathematics, especially geometry. These instructions for building fire altars using ropes include constructions of squares and rectangles, along with transformations of one shape to another with the same area. Using GeoGebra applets and hands-on activities, students can explore some mathematical contributions from ancient India, and strengthen their own geometric reasoning. For more of a challenge, students can prove that the instructions are correct, resulting in the desired shape.

Figure 5. Model of a falcon fire altar from an Athirathram ceremony in 2011. (Photo from Wikimedia Commons attributed to Arayilpdas at Malayalam Wikipedia.)
References and Resources
Common Core State Standards Initiative: http://www.corestandards.org/
[Datta] Datta, Bibhutibhusan. The Science of the Sulbas: A Study in Early Hindu Geometry. Calcutta University Press, 1932.
GeoGebra: http://www.geogebra.org
[Huffman and Thuong] Huffman, Cynthia J. and Scott V. Thuong, "Ancient Indian Rope Geometry in the Classroom," Convergence (October 2015), DOI:10.4169/convergence20151001: https://www.maa.org/press/periodicals/convergence/ancient-indian-rope-geometry-in-the-classroom-mathematics-in-ancient-india
[Plofker1] Plofker, Kim. Mathematics in India. Chapter 4 in Katz, Victor, editor. The Mathematics of Egypt, Mesopotamia, China, India, and Islam: A Sourcebook. Princeton University Press, 2007, pp. 385-514.
[Plofker2] Plofker, Kim. Mathematics in India. Princeton University Press, 2009.
[Prasoon] Prasoon, Shrikant. Indian Scriptures. Pustak Mahal, 2010, Ch. 2.
[Sen & Bag] Sen, S.N., and A.K. Bag. The Śulbasūtras. Indian National Science Academy, 1983.
Acknowledgements
The authors are indebted to the editor and anonymous referees for help in improving the article. Thank you to Dr. Janet Beery for suggesting we take a look at Indian rope geometry in the first place.
About the Authors
 |
Cynthia J. Huffman is a University Professor in the Department of Mathematics at Pittsburg State University. She has always been interested in history of mathematics but her interest was especially sparked by participation in several of the MAA Study Tours. Her research areas include computational commutative algebra and history of mathematics. Dr. Huffman is a handbell soloist and has a black belt in Chinese Kenpo karate. |
 |
Scott V. Thuong is an Assistant Professor in the Department of Mathematics at Pittsburg State University. His research areas include topology, geometry, and the history of mathematics. In his spare time, Dr. Thuong enjoys a good game of badminton, table tennis, or tennis. |